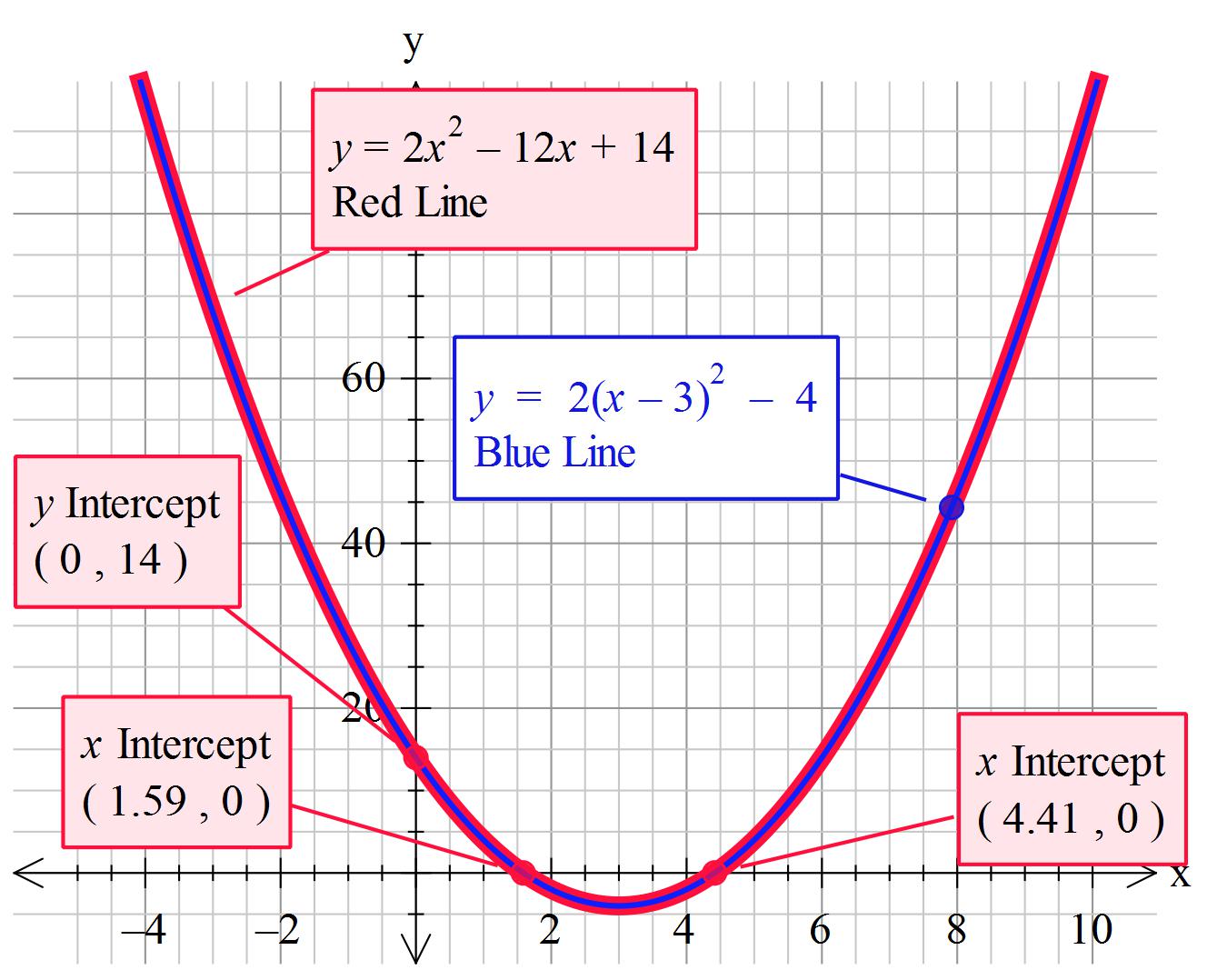
How do you solve #2x^2-12x=-14# by completing the square?

Write as #2x^2-12x+14=0#

#color(red)("Method for completing the square in detail")#

#color(blue)("Completing the square")#

What process we are about to do introduces a value that is not in the original equation. So we mathematically compensate for this by the inclusion of a correction value. This correction value would turn the introduced error into 0 if we were to carry out the addition.

Suppose we had #2z+56#. Then suppose we added 4 which is viewed as an error. However if we write #2z+56+4-4# we have corrected the error

Let #k# be the constant of correction

Write as #" "2(x^2-6x)+14+k=0#

Move the power of 2 to outside the bracket

#" "2(x-6x)^2+14+k=0#

divide the 6 from #6x# by 2

#" "2(x-6/2 x)^2+14+k=0#

Discard the #x# from #6/2#

#" "2(x-6/2)^2+14+k=0#
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
The error comes from the #-6/2# This is inside a bracket that is squared and then multiplied by the 2 outside the bracket

So the error is #2xx(-6/2)^2 = +36/2=+18#

This has to be turned into 0 by #k# so #" "k+18=0 =>k=-18#
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

#color(brown)(2(x-12/2)^2+14+k=0)color(green)(""->" "2(x-6/2)^2+14-18=0)#

#" "color(blue)(bar(underline(|" "2(x-3)^2-4=0" "|)))#
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
#color(blue)("Determine x intercepts")#

Write as:# " "(x-3)^2=4/2#

Square root both sides

#" "x-3=+-sqrt(2)#

#" "x=+3+-sqrt(2)#

#color(blue)(" "x~~4.414" and "x~~1.586" to 3 decimal places")#

Completing the square is based on the consistency of the answers to the square of a binomial.

#(x - 3)^2 = x^2 - 6x + 9#
#(x - 5)^2 = x^2 - 10x + 25#
#(x + 6)^2 = x^2 + 12x + 36#
In all of the products above, #ax^2+ bx + c# we see the following:

#a = 1#
The first and last terms, #a and c# are perfect squares.'
There is a specific relationship between 'b' - the coefficient of the #x# term and 'c'. Half of b, squared equals c.

Knowing this, it is always possible to add in a missing value for #c# (ie complete the square) to make the square of a binomial, which can then be written as #(x+?)^2#

In #2x^2 -12x = -14#, -14 has already been moved to the right hand side. The equation must be divided by 2 to make #a# = 1

This gives:

#x^2 -6x = -7#

NOW the correct value of #c# can be added to BOTH sides of the equation to give the answer to the square of a binomial.

#x^2 - 6x + color(red)(9)# = #-7 + color(red)(9) rArr [9 = (-6÷2)^2]#
#(x - 3)^2 = 2 rArr# where -3 is from #-6÷2#
#x - 3 = +-sqrt2 rArr# take the square root of both sides

This gives 2 possible answers for #x#.

#x = sqrt2 + 3# OR #x = -sqrt2 + 3#