How do you write an equation of #y=cosx# with 3 units to the left and pi units up?

Question

4967 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-06-18T13:20:29

Add 3 to the argument of the cosine function and add #pi# after the cosine function.

Here is a graph of the equation #y = cos(x)#:

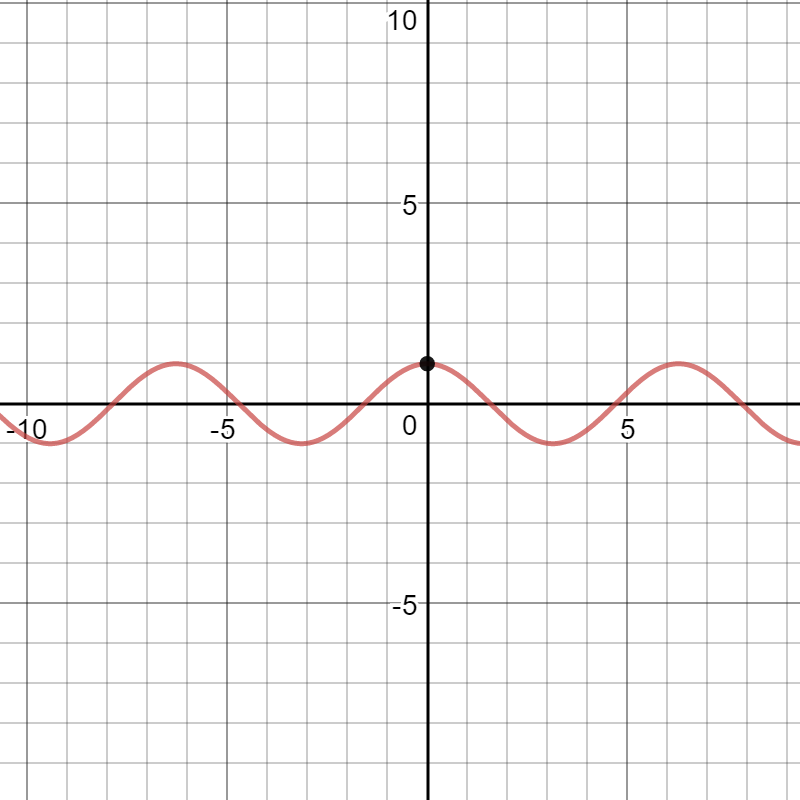
Desmon.com

Please observe that I have placed a dot at the point were #x=0#

We want to change the equation so that point is at x = -3. Lets add a constant, c, to the argument of the cosine function.

#y = cos(x+ c)#

We want #x+c# to equal 0 when #x=3/2pi#

Here is the equation for x plus c equals zero:

#x+c = 0#

Here is the equation forcing x to be -3:

#-3+c = 0#

We can solve for d:

#c = 3#

This makes the equation become:

#y = cos(x+3)#

Here is the graph for the equation:

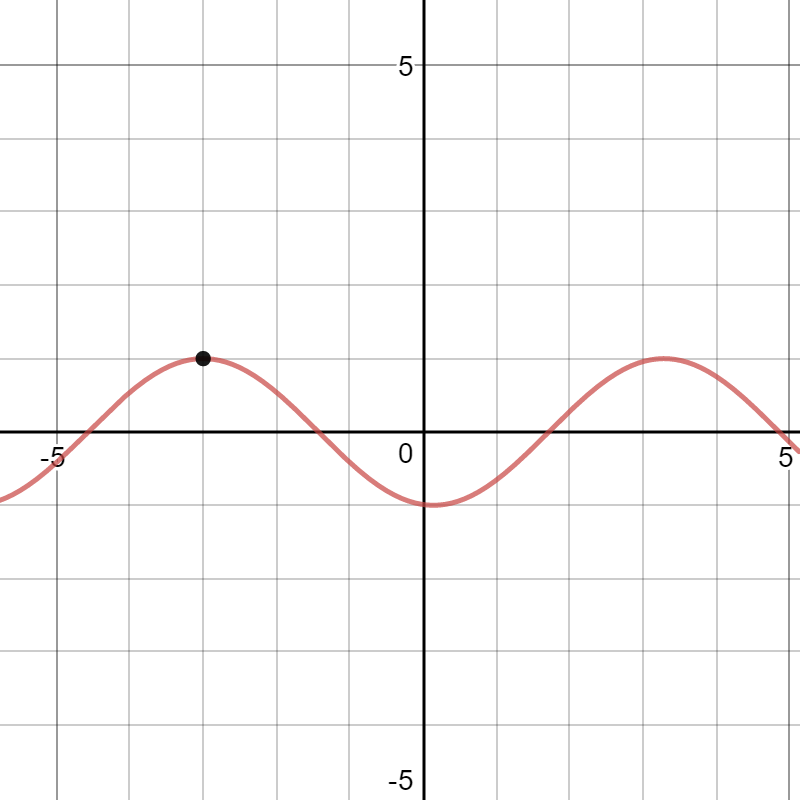
Desmos.com

Please observe that the cosine function has shifted 3 units to the left as requested.

Let's add another constant, d, to the equation:

#y = cos(x+3)+d#

We the cosine function is 1, we want y to equal #pi + 1#.

Here is the equation for that:

#pi+1 = 1 + d#

Solve for d:

#d = pi#

Here is a graph of the equation #y = cos(x+3)+pi#:
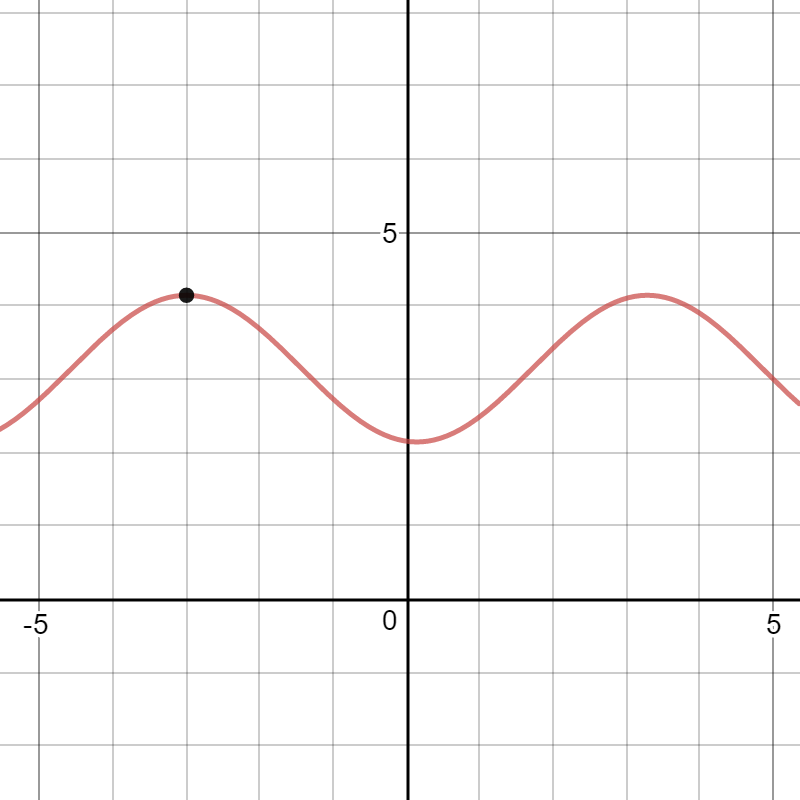
Desmos.com

Please observe that I have shifted the curve 3 units to the left and up #pi# units.