How do you solve #-(3k-12)=48# using the distributive property?

Question

3097 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-05-03T23:48:46

#k = -12#

We first simplify the left hand side using the distributive property, shown here:
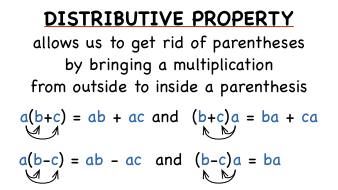
cdn.virtualnerd.com

Following this image, the left hand side of the equation would become:
#-1 * 3k - 1 * -12#

#-3k + 12#

Putting this back into the equation:
#-3k + 12 = 48#

Now, subtract #color(blue)12# from both sides of the equation:
#-3k + 12 quadcolor(blue)(-quad12) = 48 quadcolor(blue)(-quad12)#

#-3k = 36#

Divide both sides by #color(blue)(-3)#:
#(-3k)/color(blue)(-3) = 36/color(blue)(-3)#:

So:
#k = -12#

Hope this helps!