How do you graph and list the amplitude, period, phase shift for #y=-sin(x-pi)#?

1 Answer
Shell
Sep 9, 2016

Use the form #y=Asin(Bx-C)+D#

Explanation:

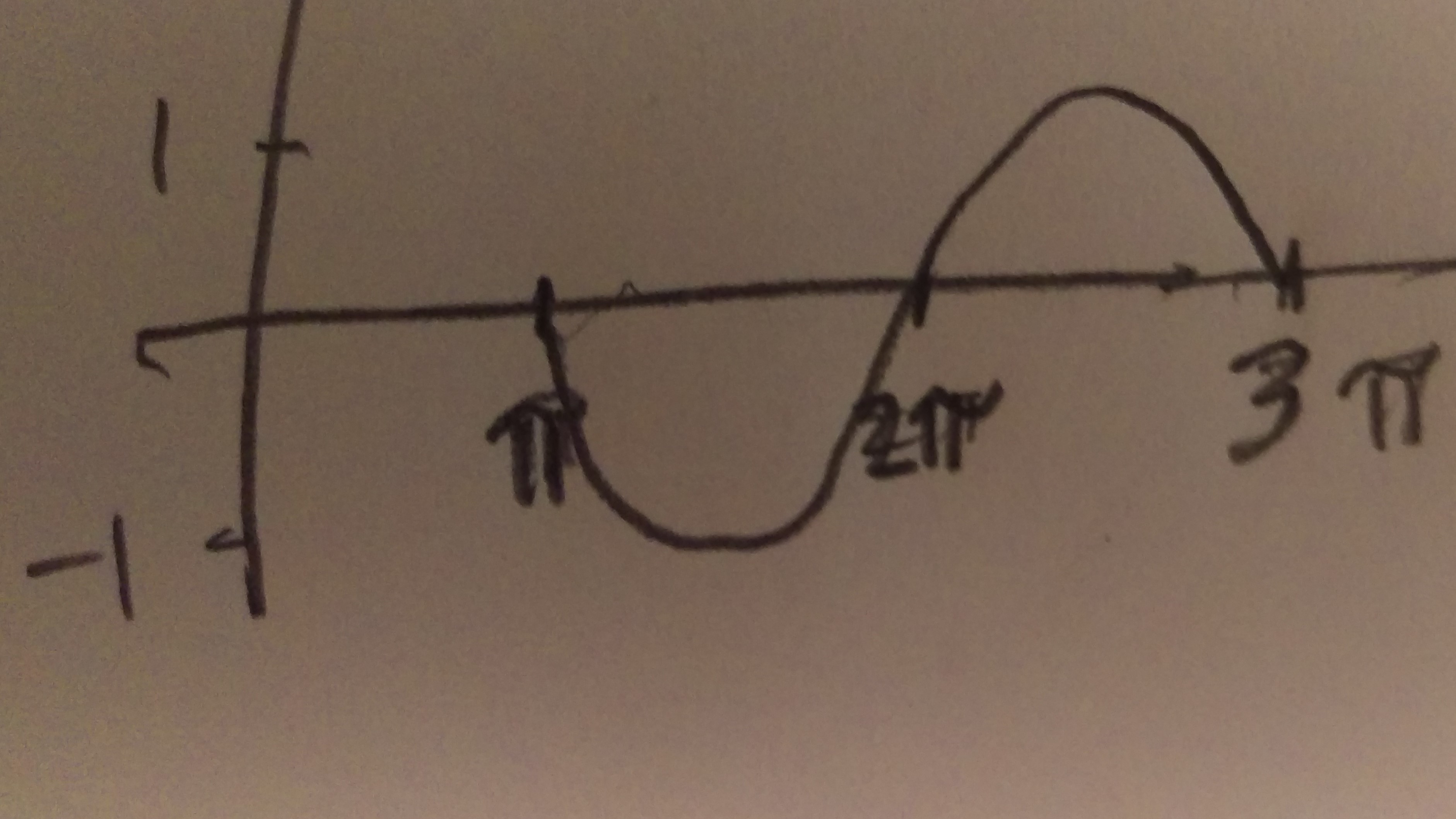
#y=Asin(Bx-C)+D#

#abs(A)# = amplitude
#(2pi)/B# = period
#C/B# = phase shift
#D# = vertical shift

#y=-sin(x-pi)#

In this example, #A=-1# and #absA = 1# so the amplitude is 1.

Period = #(2pi)/B = (2pi)/1 = 2pi#

Phase shift = #C/B = pi/1=pi# to the right. A negative sign in the parentheses means shift right.

To graph, one complete sine curve must be #2pi# "wide" because the period is #2pi#. And, a phase shift of #pi# to the right means the beginning of the sine curve should start at #pi#. Also, note the negative in front of the sin. That means the graph should be flipped over the x axis.

enter image source here

Related questions
See all questions in Translating Sine and Cosine Functions
Impact of this question
6691 views around the world
You can reuse this answer
Creative Commons License