Trigonometric form is where
#a + bi = absz(cosx + isinx)#.
#absz# is given by Pythagoras', #sqrt(a^2+b^2)#, using the values from #a + bi#.
#a + bi = 0 - 6i#
#sqrt(a^2+b^2) = sqrt(0^2 + (-6)^2) = sqrt36 = 6#
Now you can divide both sides by #6#, so
#(-6i)/6 = (6(cosx + isinx))/6#
#-i = cosx + isinx#
This gives us, obviously, that
#cosx = 0#
#sinx = -1#
and so, superimposing the graphs and finding points that satisfy the simultaneous equations,
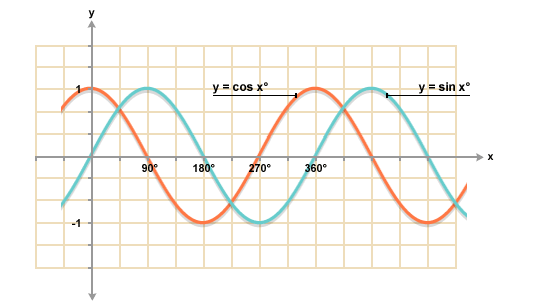
At the point #x = 270#, the #cosx# graph is #0# and the #sinx# graph is at #-1#, which satisfies the equations. Being repeating patterns, obviously there will be other results, such as #x = -450, -90, 630, 990# etc.
Putting all of this back together,
#-6i = 6(cos270 + isin270)#
or
#-6i = 6(cos((3pi)/2) + isin((3pi)/2))#


