By considering the roots of #z^5 =1#, how do you show that cos(2π/5) + cos(4π/5) + cos(6π/5) + cos(8π/5) = -1?

Question

20901 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-02-25T08:33:20

Please see below.

As #z^5=1=(cos0+isin0)#

According to De-Moivre's theorem

#z=(z^5)^(1/5)=(cos((2npi)/5)+isin((2npi)/5))# and we get five roots of #1# by changing value of #n# from #0# to #4# and we get five roots as

#cos0+isin0#or #1+i0#, #cos((2pi)/5)+sin((2pi)/5)#, #cos((4pi)/5)+sin((4pi)/5)#, #cos((6pi)/5)+sin((6pi)/5)# and #cos((8pi)/5)+sin((8pi)/5)#

and these five roots, when graphed on the complex plane, are equally spaced around a circle of unit radius as shown below.

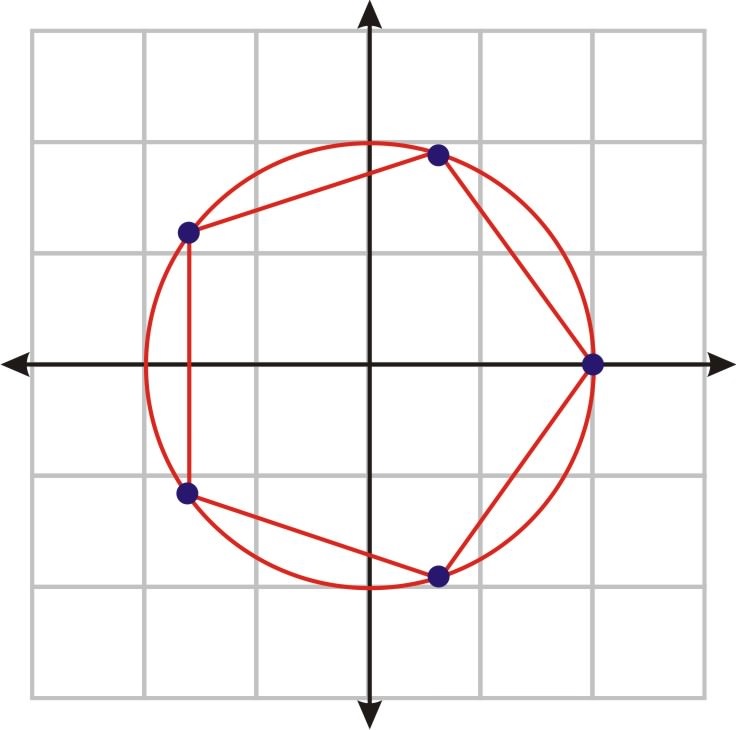
enter image source here

and it is apparent that their sum will be zero.

As such comparing real and imaginary parts we should have

#1+cos((2pi)/5)+cos((4pi)/5)+cos((6pi)/5)+cos((8pi)/5)=0#

and #0+sin((2pi)/5)+sin((4pi)/5)+sin((6pi)/5)+sin((8pi)/5)=0#

i.e. #cos((2pi)/5)+cos((4pi)/5)+cos((6pi)/5)+cos((8pi)/5)=-1#

and #sin((2pi)/5)+sin((4pi)/5)+sin((6pi)/5)+sin((8pi)/5)=0#