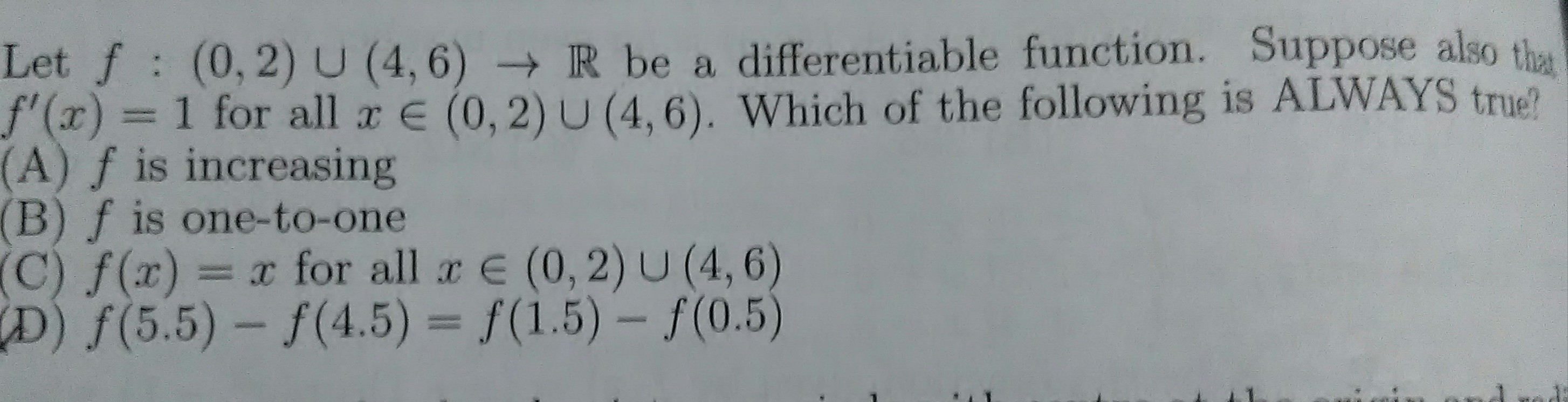f is differentiable on each of (0,2) and (4,6).
Since differentiability implies continuity, f is also continuous on each of the open intervals.
So, for any interval [a,b] entirely within one of these open intervals, we can apply the Mean Value Theorem
f is continuous on [a,b] and
f is differentiable on (a,b)
Therefore, there is a c in (a,b) such that
f(b)-f(a) = f'(c)(b-a).
Furthermore, since f'(c) = 1, we see that
So, for any interval [a,b] entirely within one of these open intervals,
f(b)-f(a) = (b-a).
Therefore,
f(5.5)-f(4.5) = 1 = f(1.5)-f(0.5)
The function
f(x) = {(x+2,"if",0 < x < 2),(x-2,"if",4 < x < 6):} satisfies the given condition but fails A, B, and C. The graph is shown below.