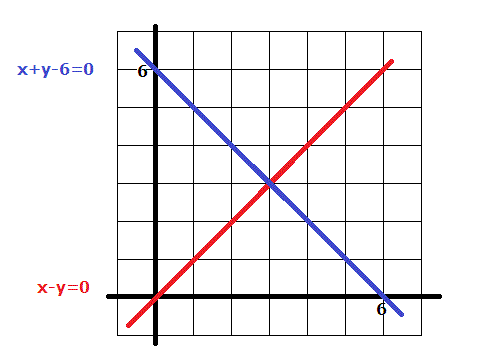
How do you solve the system #x + y - 6 = 0# and #x - y = 0# by graphing?

1 Answer
Alan P.
Jan 14, 2016

Draw the lines for the linear equations given and note the point at which the two line insect (in this case at #(x,y) = (3,3)# ).

Explanation:

The easiest points to use for graphing purposes are often the intercepts.

For #color(blue)(x+y-6=0)#
the intercepts are at #(color(blue)(0,6) )# and #(color(blue)(6,0))#

For #color(red)(x-y=0)#
the x and y-intercepts are the same point: #(color(red)(0,0))#
so it will be necessary to pick one more point;
since we already have a #6# lets pick #x=6# which given the equation implies #y=6# and we have a second point #(color(red)(6,6))#

Drawing a line connecting
#color(white)("XXX")(color(blue)(0,6))# and #(color(blue)(6,0))#
and another connecting
#color(white)("XXX")(color(red)(0,0)# and #(color(red)(6,6))#
gives us a point of intersection at #(color(green)(3,3))#
enter image source here

Related questions
See all questions in Graphs of Linear Systems
Impact of this question
10070 views around the world
You can reuse this answer
Creative Commons License