Divide each segment of the system of inequalities by #color(red)(2)# to solve for #x# while keeping the system balanced:
#-3/color(red)(2) < (2x)/color(red)(2) <= 6/color(red)(2)#
#-3/2 < (color(red)(cancel(color(blac)(2)))x)/cancel(color(red)(2)) <= 3#
#-3/2 < x <= 3#
Or
#x > -3/2#; #x <= 3#
Or, in interval notation:
#(-3/2, 3]#
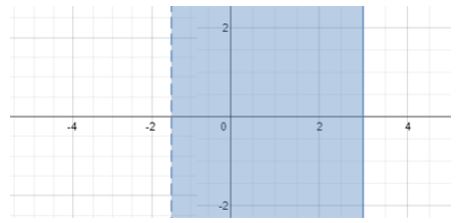
To graph this we will draw vertical line at #-3/2# and #3# on the horizontal axis.
The line at #-3/2# will be a dashed line because the inequality operator does not contain an "or equal to" clause. This indicates #-3/2# is not part of the solution set.
The line at #3# will be a solid line because the inequality operator contains an "or equal to" clause. This indicates #3# is part of the solution.
We will shade between the two lines to show the solution set.