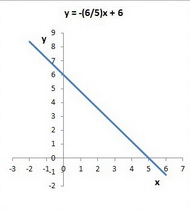
How do you find the x and y intercept and slope of #y= - 6/5x + 6#?

Question

2014 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2015-07-10T22:38:56

The slope is #-6/5#, the #y#-intercept is at (#0,6#), and the #x#-intercept is at (#5,0#).

The "slope-intercept" form of a straight line equation is

#y = mx + b#,

where #m# is the slope and #b# is the #y#-intercept.

Your equation is

#y = -6/5x + 6#

If we compare the two equations, we see that

#m = -6/5# and #b = 6#.

So the slope is #-6/5# and the #y#-intercept is at (#0,6#).

To get the #x#-intercept, we set #y = 0# and solve for #x#,

#y = -6/5x + 6#

#0 = -6/5x + 6#

#0 = -6x + 30#

#6x = 30#

#x = 30/6 = 5#

The #x#-intercept is at (#5,0#).

enter image source here