How do you convert # (1, (3pi )/ 4) # into cartesian form?

1 Answer
Alan P.
Oct 25, 2016

#"Polar: "(r,theta)=(1,(3pi)/4)color(white)("XX")rarrcolor(white)("XX")color(green)("Cartesian: "(x,y)=(-1/sqrt(2),1/sqrt(2))#

Explanation:

An angle of #(3pi)/4# is equivalent to a reference angle of #pi/4# in the second Quadrant.

Here is a fairly standard image of this relationship
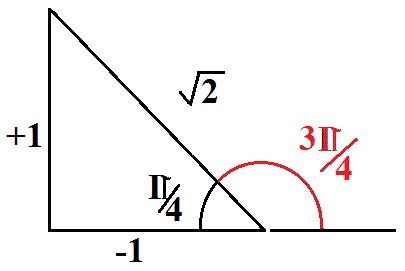
enter image source here
However the polar coordinates tell us that the radius (hypotenuse) needs to be #1#,
so all sides need to be scaled down by dividing by #sqrt(2)#
giving x-coordinate: #-1/sqrt(2)# and y-coordinate: #1/sqrt(2)#

Related questions
See all questions in Converting Equations from Polar to Rectangular
Impact of this question
2233 views around the world
You can reuse this answer
Creative Commons License