Question #9ff5f

Right from the start, you know that your solution interval cannot include #x = 1# because that is the one value of #x# that will make the denominator undefined.

So you know that you need to have #x != 1#.

Now, let's separate this compound inequality into two inequalities

#-1 < (x+1)/(x-1) " " and " " (x + 1)/(x-1) < 0#

Remember, the solution interval must satisfy both inequalities!

For the first inequality, you know that

#-1 < (x+1)/(x-1)#

Now, it is absolutely vital to keep in mind that we can have #2# possible cases here

#color(white)(a)#

• #ul(x -1 < 0)#

In this case, the first inequality can be rewritten as

#-1 * (x-1) color(red)(>) x+1#

Notice the fact that we must flip the sign of the inequality because we're multiplying on both sides by a negative number, since #x - 1 < 0#.

This simplifies to

#-x + 1 > x + 1#

#-2x > 0 implies x < 0" "color(blue)((1))#

#color(white)(a)#

• #ul(x - 1 >0)#

In this case, the first inequality can be written as

#-1 * (x-1) < (x+1)#

This simplifies to

#-x + 1 < x + 1#

#-2x < 0 implies x > 0#

However, keep in mind that in this case, we need

#x - 1 > 0 implies x > 1#

so you can say that

#x > 1" "color(blue)((2))#

So in order for the first inequality to be true, you need

# overbrace((-oo", " 0))^(color(blue)("from (1)")) " " or " " overbrace((1", " oo))^(color(blue)("from (2)"))" "color(darkorange)("( * )")#

#color(white)(a/a)#
For the second inequality, you know that

#(x+1)/(x-1) < 0#

The same approach applies here as well--you have two possible cases to look at.

• #ul(x-1 < 0)#

In this case, you have

#x + 1 color(red)(>) 0 * (x-1)#

This simplifies to

#x + 1 > 0 implies x > -1#

Since you also need

# x - 1 < 0 implies x < 1#

you can say that you have

# -1 < x < 1" "color(darkgreen)((1))#

#color(white)(a)#

• #ul(x - 1 > 0)#

In this case, the second inequality can be rewritten as

#x+ 1 < 0 * (x-1)#

This simplifies to

#x + 1 < 0 implies x < -1#

But since you also need

#x - 1 > 0 implies x > 1#

you can say that you have

#1 < x < -1 implies x in {O/}" "color(darkgreen)((2))#

According to #color(darkgreen)((1))# and #color(darkgreen)((2))#, the second inequality is only satisfied by

#x in (-1, 1)" "color(darkorange)("(* *)")#

#color(white)(a/a)#
Finally, to find the solution interval for the compound inequality, you need to intersect the #color(darkorange)("( * )")# and #color(darkorange)("(* *)")# solution intervals.

You have

#(- oo, 0) " " or " " (1, oo)" " and " " (-1, 1)#

the equivalent of

#(- oo, 0) " " uu " " (1, oo)" " nn " " (-1, 1)#

which gets you

#x in (-1, 0)#

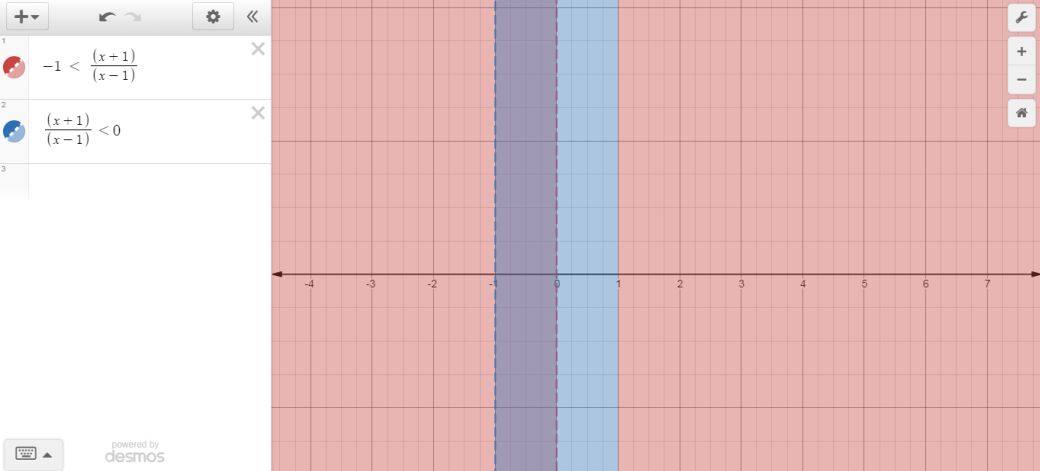
To double-check the result, you can either graph the two inequalities separately and intersect their solution intervals, or graph the compound inequality and look at the solution interval.

The graph for #-1 < (x+1)/(x -1)# looks like this

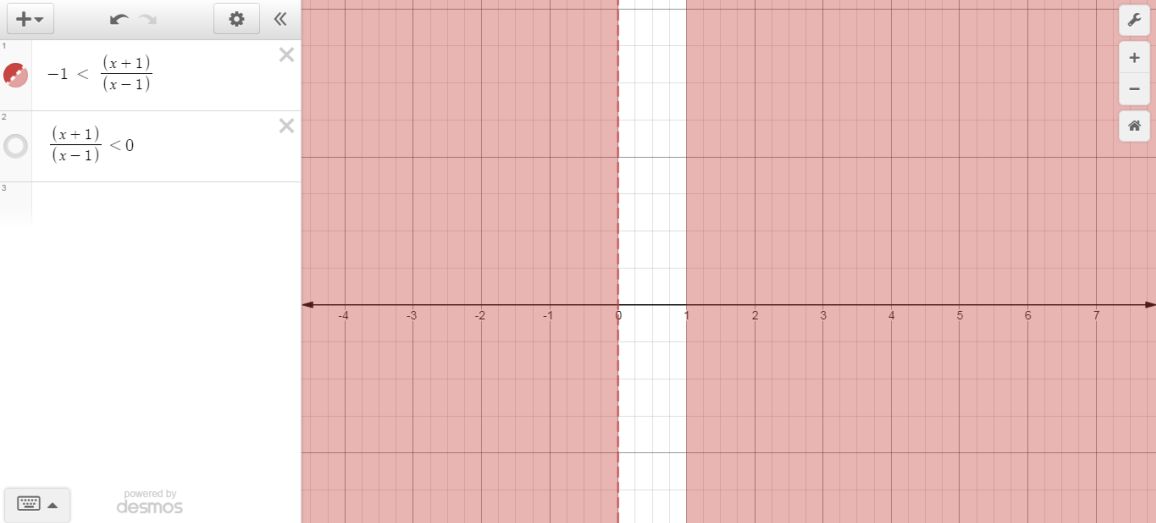
The graph for #(x+1)/(x-1) < 0# looks like this

and the graph for the compound inequality looks like this