Make the internet a better place to learn
Question #bfcde

Answer:
Yes, there is gravity in space, otherwise, all of our satellites around the Earth would float away. For that matter, Earth and the other planets would fly away from the Sun!!
Explanation:
Think about how gravity works. Objects are pulled to the center of the Earth. It's important to note its the center of the Earth, and not just "down." Now picture a cannon at the top of a huge tower. A cannon ball that is dropped from the tower will simply fall toward the base of the tower.

The cannon ball falls at a constant acceleration of 1g toward the earth, which Galileo showed is the same acceleration as everything else on the Earth! This is important to remember!!
What if the cannon ball is fired from the cannon, though? It still accelerates toward the earth at the same rate as the dropped ball, but it will move forward a bit while it falls. Because the Earth is round, the ground actually drops away from the ball! So the faster the ball is launched, the longer it will take to hit the ground.
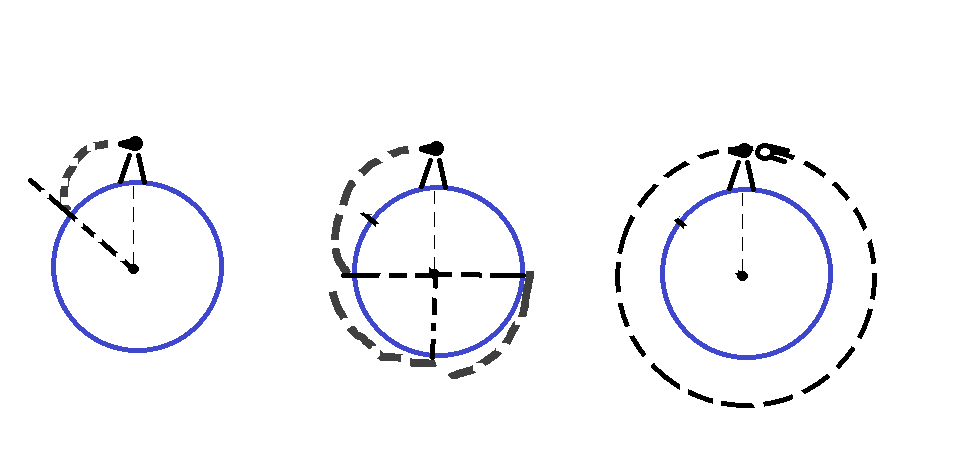
In fact, if the ball was launched forward fast enough, it would never hit the ground at all. It would travel all the way around the earth and collide with the back of the cannon! This is how an orbit is achieved! Remember though, gravity is still pulling on the cannon ball. If it wasn't, the ball would just fly off into space in a straight line. That is Newton's first law, inertia, in action.
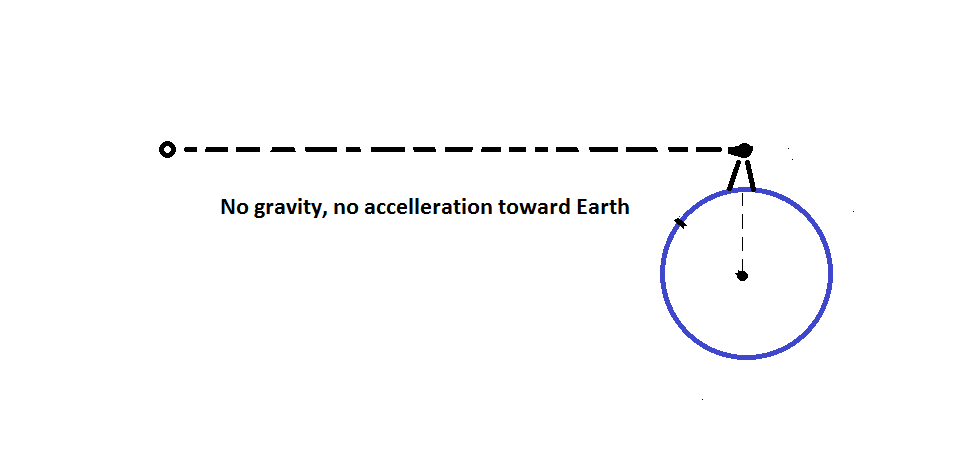
So the moon is like the cannon ball. It just moves forward as fast as it falls and therefore stays in orbit. As for the astronauts, they float in their space stations because the astronaut and the station are both falling toward the earth at the same rate. They are both in free fall.
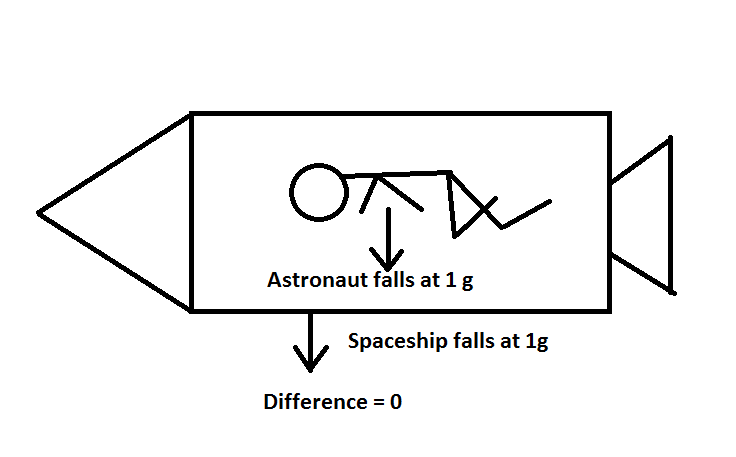
The result is that the astronaut is never able to catch up to his spaceship. This is why he floats. You can experience this yourself with a trampoline and a baseball. As you bounce, release the baseball and from your perspective, it will appear to "rise up" from you hand, at least until you bounce again.
Question #de828
Answer:
The distance between the earth and the body must be 259 358 400 m for the gravitational attraction of the sun and the earth on the body being balanced.
Explanation:
The gravitational attraction between two bodies is calculated as follows:
with
If we place a body on a straight line between the earth and the sun, the resulting gravitational attractions will be:
We are considering the situation when
Knowing that
How do astronomers use the Doppler effect to determine the velocities of astronomical objects?

Answer:
Astronomers analyze the shift of spectral patterns of the light emitted or absorbed by those objects.
Explanation:
One of the problems which prompted Einstein's work on relativity was the constant speed of light in a vacuum. Classical physics would expect that even if the emission speed of light,
Laboratory observations, however, consistently measured the speed of light to be
Since the wavelength of light determines its color, we call this change "blueshift" for objects moving toward the observer, and "redshift" for objects moving away. Edwin Hubble derived a formula for measuring velocity based on the change in wavelength.
This means that we need to know the emitted wavelength of the light in order to calculate the velocity. This is where spectroscopy comes in.
Every element on the periodic table has its own unique emission spectrum. This spectrum is formed when electrons within the atoms of these elements are excited to higher energies and then relax back to the ground state. In order to relax the atoms give off light. Because of quantum mechanics, however, electrons can only exist in specific orbital energies, so the atoms can only emit photons with wavelengths that correspond to the energies of these transitions.
Hydrogen is a convenient element to use for spectroscopy because not only does it have a fairly simple emission spectrum, it is abundant throughout the universe. If we analyze the light spectrum of a galaxy, we would expect to find an emission line at
That's
A ball is thrown towards a cliff with an initial speed of 30.0 m/s directed at an angle of 60.0◦ above the horizontal. The ball lands on the edge of the cliff 4.00 s after it is thrown. ?

Answer:
(a)
(b)
(c)
Explanation:
(a)
Diagram (a) describes the scenario. (apologies for the artwork):
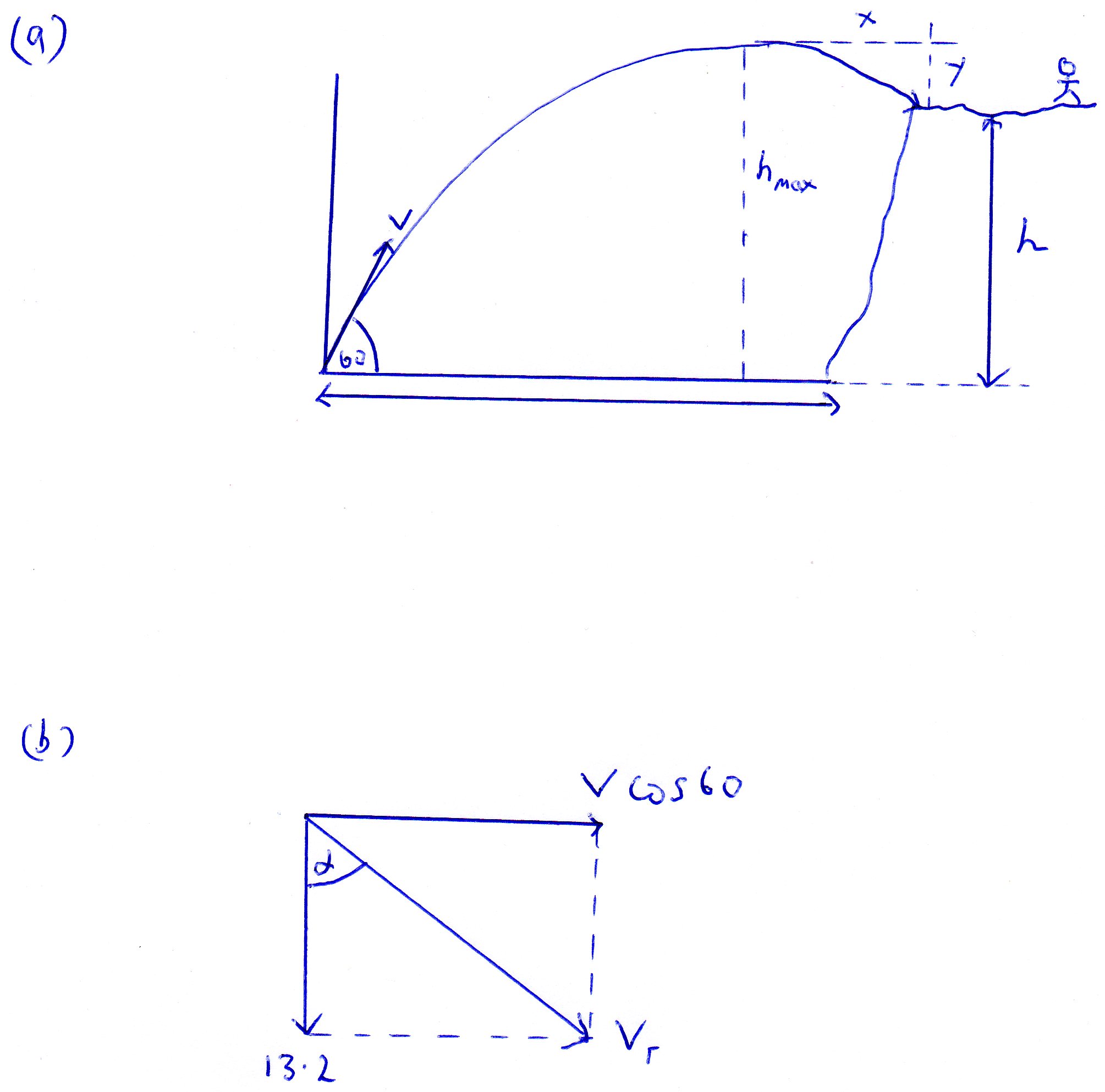
(a)
To get
The vertical component of the initial velocity
So the expression for
(b)
To get the maximum height
This becomes:
(c)
To get the vertical component
In diagram (a) the distance travelled is marked "y".
This can be found from:
So now we can say that;
Now we can use:
This becomes:
Now we know the vertical and horizontal components of velocity we can find the resultant
Using Pythagoras we can say that:
If you want the angle you can say that:
From which
Question #487bc

Disclaimer: Yes, this will be long! No getting around it!
It really helps to know the derivations. So, what do we know?
#\mathbf(dH = dU + d(PV))# (1)#\mathbf(dU = delq_"rev" + delw_"rev")# (2)#\mathbf(delw_"rev" = -PdV)# (3)#\mathbf(((delH)/(delT))_P = C_P# (4)-
#\mathbf(((delU)/(delT))_V = C_V# (5) -
An isothermal situation assumes a constant temperature during the expansion process.
- An adiabatic situation assumes no heat flow
#\mathbf(q)# contributes to the internal energy#U# . -
The volume might have changed, but we don't know how, exactly. Even though we were given
#C_V# , we can't assume that it is a constant-volume situation since we can convert from#C_V# to#C_p# pretty easily (#C_p - C_V = nR# for an ideal gas). -
The pressure decreased, i.e. it is NOT constant. That means that we should expect to eventually somehow use the relationship
#PV = nRT# .
So, having said that, let's see...
---PART A---
ENTHALPY AND INTERNAL ENERGY
In an isothermal process, we know that
#dH = C_pdT#
#int dH = color(blue)(DeltaH) = int_(T_1)^(T_2) C_pdT = color(blue)(0)#
...and using (5), we get:
#dU = C_VdT#
#int dU = color(blue)(DeltaU) = int_(T_1)^(T_2) C_VdT = color(blue)(0)#
...for an ideal gas. REMEMBER THIS:
"The energy of an ideal gas depends upon only the temperature" (McQuarrie, Ch. 19-4). In other words, when the temperature is constant, enthalpy and internal energy are both
#0# for an ideal gas.
REVERSIBLE HEAT FLOW
Now, solving for the reversible heat flow, using (1), that means
#delq_"rev" + delw_"rev" = -(PdV + VdP)#
and then using (3):
#delq_"rev" - cancel(PdV) = - cancel(PdV) - VdP#
#delq_"rev" = -VdP#
#intdelq_"rev" = -int_(P_1)^(P_2) VdP#
We don't know how the volume changed, just how the pressure changed, so we have to substitute
#color(blue)(q_"rev") = -int_(P_1)^(P_2) (nRT)/P dP#
#= -nRT int_(P_1)^(P_2) 1/P dP#
#color(blue)(= -nRT ln|(P_2)/(P_1)|)#
REVERSIBLE WORK
For reversible work, note that:
#dU = delq_"rev" + delw_"rev"#
#cancel(DeltaU)^(0) = q_"rev" + w_"rev"#
thus:
#color(blue)(w_"rev" = -q_"rev")#
---PART B---
REVERSIBLE HEAT FLOW, AND INTERNAL ENERGY
In an adiabatic process, we should know that
#dU = C_VdT = delw_"rev" = -PdV#
In this case,
#color(blue)(DeltaU) = int_(T_1)^(T_2) C_VdT#
#= color(blue)(3/2 R (T_2 - T_1))#
REVERSIBLE WORK
Next, we can use the relationship recently established to determine
#color(blue)(w_"rev" = DeltaU)#
ENTHALPY
Finally, we still need
#DeltaH = int_(T_1)^(T_2) C_pdT#
Thus:
#color(blue)(DeltaH) = C_p(T_2 - T_1)#
#= (C_V + nR)(T_2 - T_1)#
#= (3/2 R + R)(T_2 - T_1)#
#= color(blue)(5/2 R(T_2 - T_1))#
One #"mol"# of an ideal gas, initially at #"300 K"#, is cooled at constant #"V"# so that #"P"_f# is #1/4 "P"_i#. Then the gas expands at constant #"P"# until it reaches #"T"_i# again. What is the work #w# done on the gas?

DISCLAIMER: Yes, this will be long!
This is one of those problems where drawing a "P-V diagram" would really help. Let's see approximately what that looks like for this problem.
We know that:
STEP 1
STEP 2
From that, we construct the PV-diagram as follows:
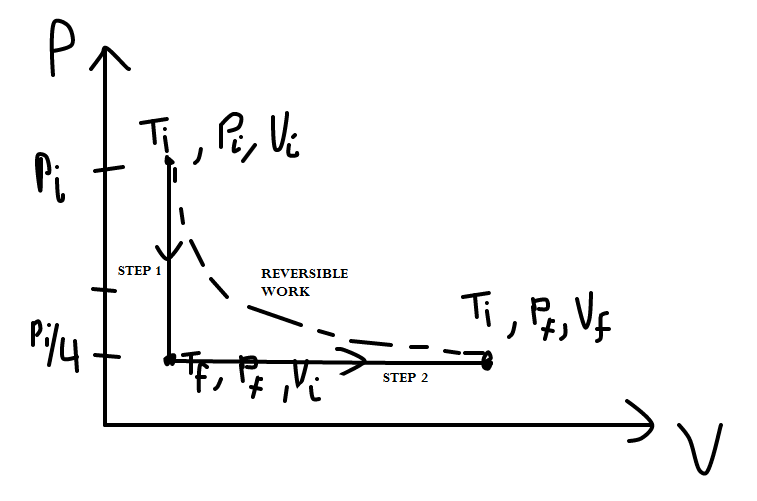
Notice how we have the possibility for a reversible work process that accomplishes the same thing more efficiently.
TWO-STEP PATH: STEP 1
We should know that inexact differential work
STEP 1: WORK
We can then proceed by noting that
#color(blue)(w_1) = - int PdV = color(blue)(0)#
STEP 1: HEAT FLOW
Since PV-work didn't happen here, it was all heat flow, conducting out through the surfaces of the container, slowly leading the gas particles to redistribute more loosely without changing in volume, thus naturally cooling the gas.
In other words, the change in internal energy was all due to heat flow at a constant volume
#color(blue)(DeltaU = q_V = ???)#
Unfortunately we don't know enough to determine
#\mathbf(DeltaU = q_V#
#\mathbf(= int_(T_1)^(T_2) C_v dT)#
Either way, this release of thermal energy means that the first work step was inefficient; thermal energy escaped the container while the work
TWO-STEP PATH: STEP 2
Okay, so what's
STEP 2: WORK
#w_2 = -int PdV#
#color(green)(w_2) = -P int_(V_i)^(V_f) dV#
#= -P(V_f - V_i)#
We don't know how the volume changed, but using the ideal gas law, we can fidget with this:
#= -cancel(P)((nRT_f)/cancel(P) - (nRT_i)/cancel(P))#
#= color(green)(-nR(T_f - T_i))#
STEP 2: TEMPERATURE
Alright, so we know
#PV = nRT#
#P_icancel(V_i)^("constant") = cancel(nR)^("constant")T_i#
#P_fcancel(V_i)^("constant") = cancel(nR)^("constant")T_f#
#P_i/T_i = P_f/T_f#
The temperature from step 1 we want is
#T_f = (P_f)/(P_i)xxT_i = 1/4 xx 300 = color(green)("75 K")#
Thus, in step 2, the gas heated from
#color(blue)(w_2 = -"1870.76 J")#
In expansion work, work is done by the gas, so it should be negative.
REVERSIBLE WORK PATH
Now, if we try that reversible path, we assume that steps 1 and 2 aren't there and that this is an efficient path where the gas is acted upon very slowly so that it has time to adjust. We know that in this path,
REVERSIBLE WORK PATH: WORK
#w_"rev" = -int PdV#
#= -int (nRT)/V dV#
#color(green)(w_"rev") = -nRT int_(V_i)^(V_f) 1/V dV#
#= color(green)(-nRTln|(V_f)/(V_i)|)#
Notice how the negative natural logarithm approximates the shape of the reversible path.
REVERSIBLE WORK PATH: VOLUME
Okay, but how did the volume change? Inversely proportionally to the pressure! The volume increased at a constant temperature, so the pressure should decrease.
Using the same trick with the ideal gas law as before:
#color(green)(w_"rev") = -nRTln|(cancel(nRT)/P_f)/(cancel(nRT)/P_i)|#
#= -nRTln|(P_i)/(P_f)|#
#= -nRTln|(P_i)/(1/4 P_i)|#
#= color(green)(-nRTln4)#
So, with
#color(blue)(w_"rev" = -"3457.89 J")#
In expansion work, work is done by the gas, so it should be negative.
A ball with a mass of # 5 kg# is rolling at #7 m/s# and elastically collides with a resting ball with a mass of #4 kg#. What are the post-collision velocities of the balls?

Answer:
I found:
Explanation:
We can use conservation of momentum
So:
Being an elastic collision also kinetic energy
So:
We can use the two equations together and we get:
From the first equation:
Substitute into the second and get:
Corresponding to:
A charge of #7 C# is at the origin. How much energy would be applied to or released from a # 5 C# charge if it is moved from # (-3 , 7 ) # to #(-7 ,-2 ) #?

Answer:
Explanation:
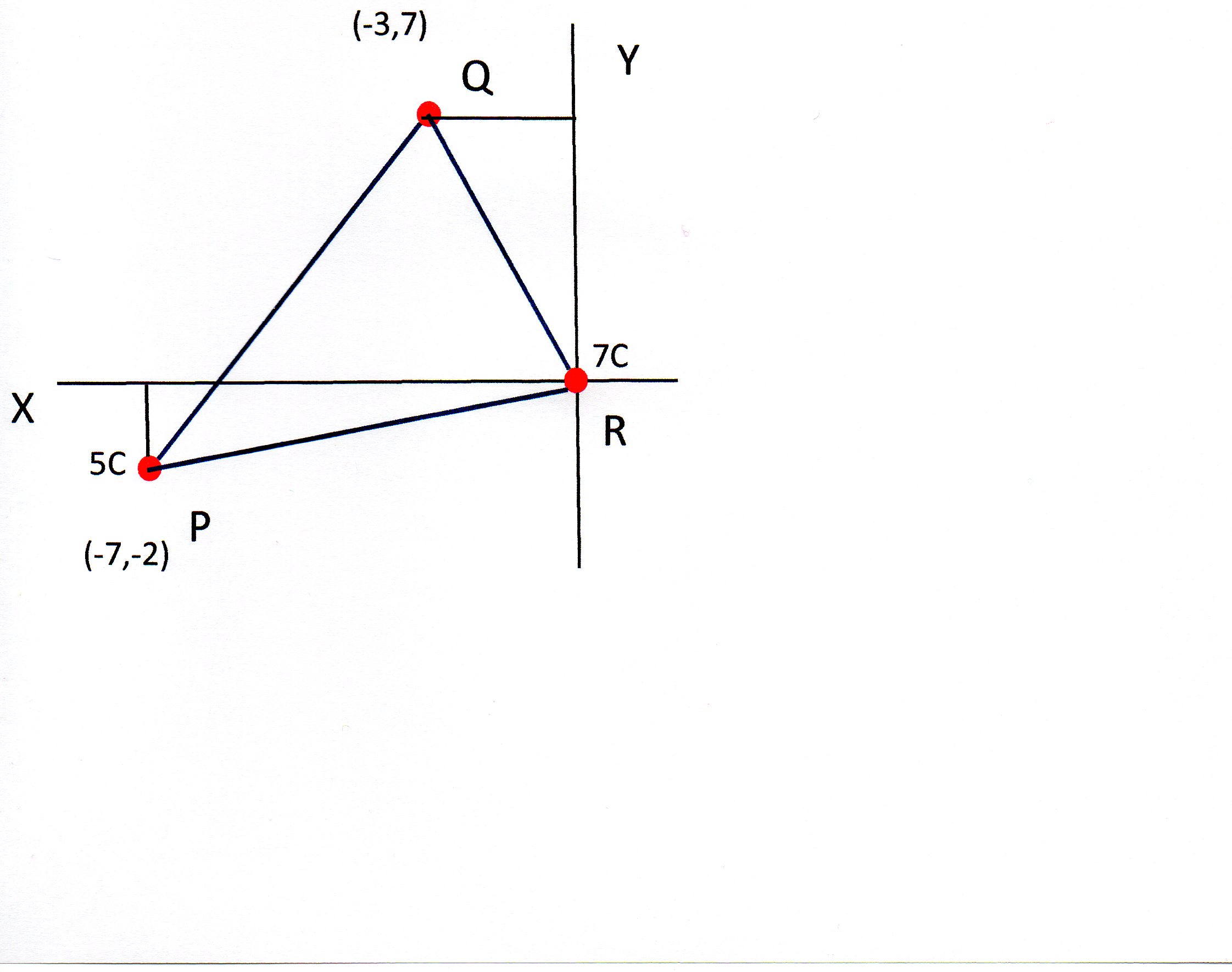
I'll work out the electric potential at points P and Q.
Then I will use this to work out the potential difference between the 2 points.
This is the work done by moving a unit charge between the 2 points.
The work done in moving a 5C charge between P and Q can therefore be found by multiplying the potential difference by 5.
We need to find the length of PR and PQ which can be done using Pythagoras:
and:
The electric potential due to a charge
So the potential at point
The potential at
So the potential difference is given by:
So the work done in moving a 5C charge between these 2 points is given by:
This is the work done on the charge.
There are no units of distance given. If this was in meters then
A projectile is shot from the ground at an angle of #(5 pi)/12 # and a speed of #3 m/s#. Factoring in both horizontal and vertical movement, what will the projectile's distance from the starting point be when it reaches its maximum height?

Answer:
Explanation:
We should start by breaking down the initial velocity into its
Note that the units are
The acceleration due to gravity is
So the projectile takes
The projectile will move about
If a #13 kg# object moving at #7 m/s# slows down to a halt after moving #10 m#, what is the friction coefficient of the surface that the object was moving over?
Answer:
Explanation:
I will show you 2 different methods to do this question :
1. Method 1 - Using Newton's Laws and Equations of Motion :
The acceleration of the object is uniform and in 1 direction and can be found from a relevant equation of motion for constant acceleration in 1 dimension as follows :
This is acceleration is caused by the frictional force which is the resultant force acting on the object and so by Newton 2 and definition of frictional forces we get :
But
2. Method 2 - Using energy considerations :
The Work-Energy Theorem states that the work done by the resultant force equals the change in kinetic energy brought about.