Buffer or no buffer? #"45 mL"# of #"0.60 M"# #"KF"# reacts with #"25 mL"# of #"0.60 M"# #"HClO"_4# to yield #"HF"# and #"KClO"_4#, all aqueous.

Yes. More info up above.

A buffer is a weak acid/base plus its salt. Like a mixture of acetic acid and sodium acetate.

#HClO_4# is a strong acid

KF is not a salt of this acid either

A buffer is a weak acid/base plus its soluble conjugate base/acid (which usually works well with an alkali metal as the cation).

The pKa of #HClO_4# is about -10, while the pKa of #F^-# is somewhere higher than 3.17, the pKa of #HF#. The pKas are not close enough that they are both considered weak acids and bases relative to each other; their #K_as# are over 13 orders of magnitude apart. In fact, it's more likely that #KF# will exist in solution as #F^-#, while #HClO_4# donates a proton to form #HF#.

In the previous sense, #HF# and #F^-# end up forming a buffer, which, as stated on the answer above, has a pH of 3.04, which reflects the pKa of HF of 3.17. #HClO_4# protonates the ionized #F^-# from #KF# and the buffer is formed then. It's kind of a tricky question.

Yes, this is a buffer with pH = 3.04.

#"KF"# is the salt of a strong base and the weak acid #"HF"#.

The #"F"^-# ion is basic and will react completely with a strong acid like #"HClO"_4#.

The #color(red)("molecular equation")# is

#"KF(aq)" + "HClO"_4"(aq)" → "HF(aq)" + "KClO"_4"(aq)"#

The #color(red)("ionic equation"# is

#"K"^+"(aq)" + "F"^(-)"(aq)" + "H"_3"O"^+"(aq)" + "ClO"_4^(-)"(aq)" → "HF(aq)" + "H"_2"O(l)" + "K"^+"(aq)" + "ClO"_4^(-)"(aq)"#

The #color(red)("net ionic equation"# is

#"F"^(-)"(aq)" + "H"_3"O"^+"(aq)" → "HF(aq)" + "H"_2"O(l)"#

The first problem is to figure out how much #"F"^-# reacts and how much is unreacted.

#"Moles of F"^(-) = 0.045 cancel("L") × "0.60 mol"/(1 cancel("L")) = "0.027 mol"#

#"Moles of H"_3"O"^+ = 0.025 cancel("L") × "0.60 mol"/(1 cancel("L")) = "0.0015 mol"#

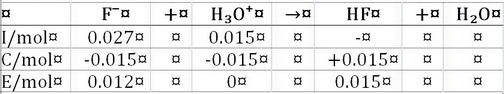
We have a buffer, because we have a solution of a weak acid #"HF"# and its conjugate base #"F"^-#.

To calculate the pH, we use the Henderson-Hasselbalch Equation.

#"HF" + "H"_2"O" → "H"_3"O"⁺ + "F"^-#; #"p"K_"a" = 3.14#

#"pH" = "p"K_"a" + log(("[F"^(-)"]")/"[HF]") = 3.14 + log((0.012 cancel("mol"))/(0.015 cancel("mol"))) = 3.14 – 0.097 = 3.04#

Note that the ratio of the concentrations is the same as the ratio of the moles, because both species are in the same solution.