Why is the empirical rule important?
1 Answer
The empirical rule, which states that nearly all data will fall within three standard deviations of the mean, can be useful in a few ways.
Explanation:
The empirical rule tells us about the distribution of data from a normally distributed population. It states that ~68% of the data fall within one standard deviation of the mean, ~95% of the data fall within two standard deviations, and ~99.7% of all data is within three standard deviations from the mean.
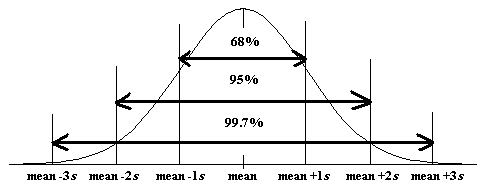
You can use the empirical rule to determine if your dataset follows a normal distribution . If you're given the mean and standard deviation of a normally distributed population, you can also determine what the probability is of certain data occurring .
For example, if the mean of a normally distributed data set is 56 and the standard deviation is 10, you know that 68% of your data will fall between 46 and 66 (one standard deviation below and above the average).
You can read more about the empirical rule here and see a slightly more complicated example of it being used here.

