Why does ammonia have 4 shells that are sp3 hybridized, rather than having the s-shell for the non-bonding electron pair and the remaining 3 p-shells used for the covalent bonding with the hydrogen atoms?

Since all three hydrogens are identical, but four electron groups are present in #NH_3#, nitrogen needed four identical orbitals to bond.

AMMONIA LOOKS OFF WITH PURE ORBITALS

Suppose we did have one #s# orbital holding the lone pair of electrons and three #p# orbitals holding #"N"-"H"# bonding-electron pairs.

That would actually imply that #"NH"_3# can only look like this, with all #2p# orbitals aligned along the cartesian axes:

but we know that's not right.

RECONCILING THE NOTION OF WHAT AMMONIA LOOKS LIKE

Here are the premises we're working with:

1. Nitrogen has its #2s#, #2p_x#, #2p_y#, and #2p_z# orbitals to use.
2. Its #2p_x# orbital is aligned precisely along the #x# axis.
3. Its #2p_y# orbital is aligned precisely along the #y# axis.
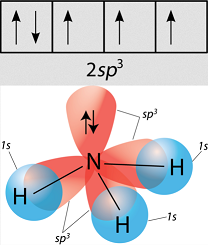
4. Its #2p_z# orbital is aligned precisely along the #z# axis.
5. Since ammonia is three-dimensional, it requires #sp^3# hybridization to make its bonds. We'll prove that in a moment.

To make a bond with three identical hydrogens AND have a fourth electron group for a lone pair, we need three dimensions, AND we need to move the orbitals off the cartesian axes!

However, if any of the #2p# orbitals move off their cartesian axis, it can no longer be a pure orbital.

A pure orbital cannot be re-aligned onto a different axis without simply becoming a different orbital.

The quantum mechanical reason why these directional pure orbitals must stay on the axes for which they were defined is that they must maintain orthogonality (perpendicularity) to be physically representable with quantum mechanical equations.

EFFECT OF HYBRIDIZATION ON ORBITAL DIRECTION

NOTE: This is just a proof, so you do not need to know how to do this; just follow it and work to understand the conclusion.

One of the major effects of hybridization is that it allows directional orbitals like the #2p_y# and #2p_z# to lie in between cartesian axes by summing their vector directions.

If we represent the #2s# and #2p# orbitals as orthogonal and normalized vectors (they are all perpendicular and all their numbers are at most #1#), we could say:

• #vec(2s) = << 0,0,0 >>#
• #vec(2p_x) = << 1,0,0 >>#
• #vec(2p_y) = << 0,1,0 >>#
• #vec(2p_z) = << 0,0,1 >>#

If you perform the dot product with any two of these, you would get #<< a,b,c >>cdot<< e,f,g >> = (axxe) + (bxxf) + (cxxg) = 0#, which establishes perpendicularity.

If you hybridize only three of these pure orbitals to get #sp^2#, you can add them to get:

#vec(2s) + vec(2p_x) + vec(2p_y) = << stackrel(x)overbrace(1),stackrel(y)overbrace(1),stackrel(z)overbrace(0) >>#

which is a two-dimensional vector on the #xy#-plane. Thus, #sp^2# hybridization cannot give you a three-dimensional structure. It can, however, give you a structure with three identical bonds spread out over the #xy#-plane.

Almost there, but not quite, because we're missing the trigonal pyramidal structure (the #2p_z# orbital would hold the lone pair here). Now if you hybridize all four pure orbitals to get #sp^3# hybridization, you get:

#vec(2s) + vec(2p_x) + vec(2p_y) + vec(2p_z) = << stackrel(x)overbrace(1),stackrel(y)overbrace(1),stackrel(z)overbrace(1) >>#

which is a three-dimensional vector, and thus can be freely rotated in any direction in three dimensions and still be a valid orbital under quantum mechanical restrictions.

(These orbitals can be scaled in any dimension as well, so we're not restricted to an orbital confined within a #1xx1xx1# space. Yay!)

A NOTE ABOUT SYMMETRY

The other reason why hybridization is necessary for ammonia is that all three identical hydrogen atoms that come in to bond need to bond identically.

A #2p_x#, #2p_y#, and #2p_z# orbital are not the same orbital. They LOOK the same, but they aren't the same because they're not facing the same direction (seems dumb, but it's true).

To make the #2s#, #2p_x#, #2p_y#, and #2p_z# orbitals look and behave the same way, we have to mix them together and spit out an #sp^3# hybridized orbital to bond identically in three dimensions.

(A general chemistry textbook probably wouldn't explain why, so you don't really need to know the nitty gritty details for this caveat.)