What is the limit of #sqrt(10x^2 -7x +6)/(5x + 8)# as x approaches infinity?

In the bottom fraction #5x+8# will start to look more like #5x# as #x# gets very big.

At the same time #sqrt(10x^2-7x+6)# will start to look like #sqrt(10x^2) = sqrt(10)x# as the #x^2# term dominates the others.

Thus #sqrt(10x^2-7x+6)/(5x+8)->(sqrt(10)x)/(5x)# as #x-> oo#

#(sqrt(10)x)/(5x)=sqrt(10)/5=(sqrt2sqrt(5))/5=sqrt(2/5)#

Hence our final answer: Here is a graph of the function. As you can see it asymptotically approaches #sqrt(2/5)#

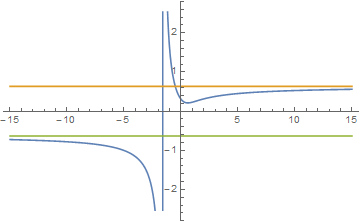
We also see that as #x# goes to negative infinity the function tends towards #-sqrt(2/5)#.

For all #x != 0#, we have

#sqrt(10x^2-7x+6) = sqrt(x^2)sqrt(10-7/x+6/x^2)#

Furthermore, #sqrt(x^2) = abs x#.

When looking for a limit as #x# increses without bound, we are concerned only with positive values of #x#, so

#lim_(xrarroo)sqrt(10x^2-7x+6)/(5x+8) = lim_(xrarroo)(sqrt(x^2)sqrt(10-7/x+6/x^2))/(x(5+8/x))#

# = lim_(xrarroo)(cancel(x)sqrt(10-7/x+6/x^2))/(cancel(x)(5+8/x))#

# = sqrt10/5#

Bonus

If we look for the limit as #x# decreases without bound, then we are concerned with negative values of #x#. For those values we have

For #x < 0#, #sqrt(x^2) = abs x=-x#.

Therefore,

#lim_(xrarr-oo)sqrt(10x^2-7x+6)/(5x+8) = lim_(xrarr-oo)(sqrt(x^2)sqrt(10-7/x+6/x^2))/(x(5+8/x))#

# = lim_(xrarr-oo)(-cancel(x)sqrt(10-7/x+6/x^2))/(cancel(x)(5+8/x))#

# = -sqrt10/5#