How do you find the limit #lim_(h->0)((2+h)^3-8)/h# ?

3 Answers
Jake M.
Mar 7, 2018

12

Explanation:

We can expand the cube:
#(2+h)^3 = 8 + 12h + 6h^2 + h^3 #

Plugging this in,

#lim_(hrightarrow 0) (8+12h+6h^2+h^3-8)/h = lim_(hrightarrow 0) (12h+6h^2+h^3)/h #

#= lim_(hrightarrow 0) (12+6h+h^2) = 12 #.

maganbhai P.
Mar 7, 2018

#12#

Explanation:

We know that,#color(red)(lim_(x->a)(x^n-a^n)/(x-a)=n*a^(n-1))#
#L=lim_(h->0)((2+h)^3-8)/h#,let,#2+h=xrArrhto0,then,xto2#
So,#L=lim_(x->2)((x^3-2^3)/(x-2))=3(2)^(3-1)=3*2^2=12#

Sunayan S
Mar 7, 2018

Image reference...

Explanation:

My notebook..

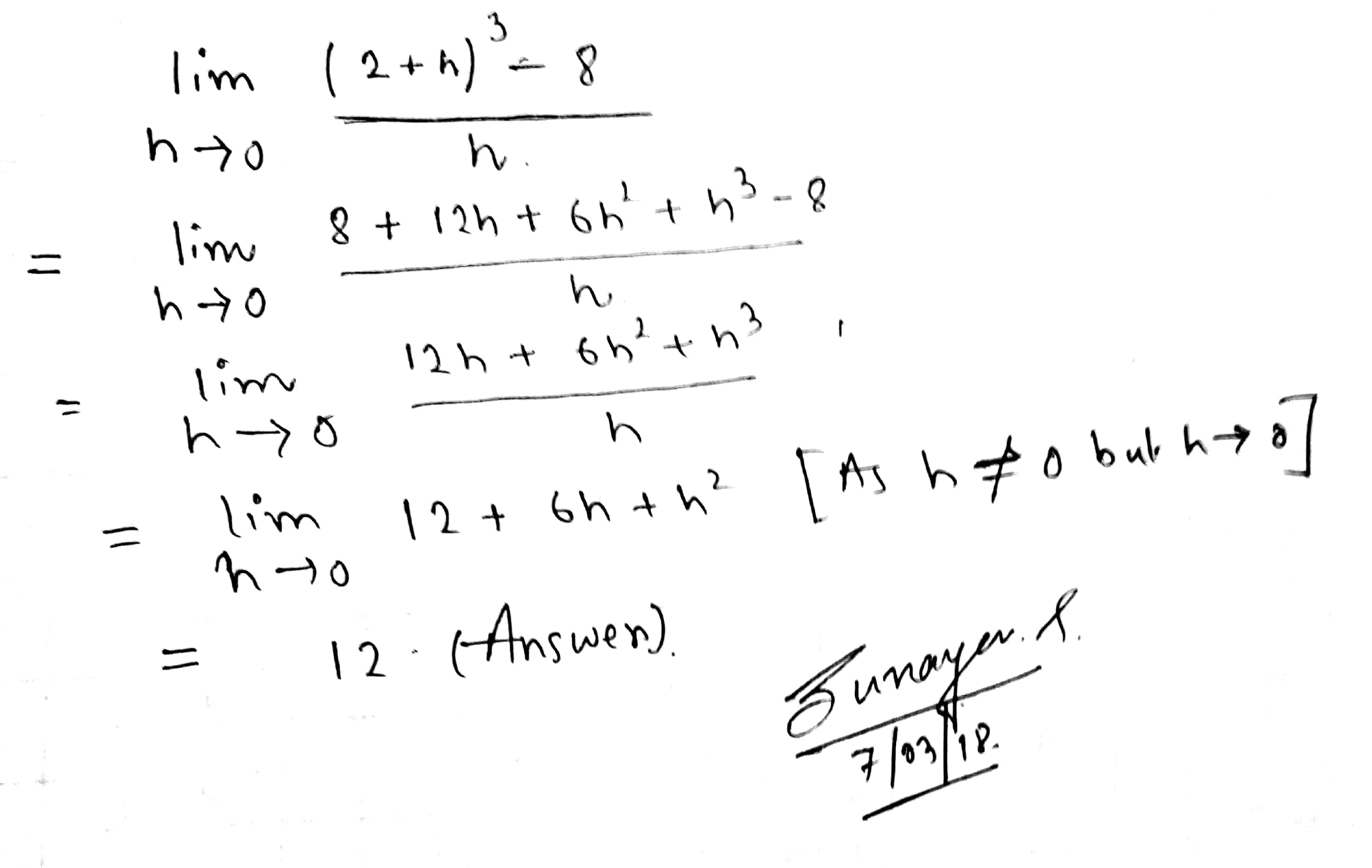
  • No intention do reply an answered answer... but as I was practicing , I added the image.
Related questions
See all questions in Determining Limits Algebraically
Impact of this question
54382 views around the world
You can reuse this answer
Creative Commons License