What is the difference between vapour pressure of a solvent and vapour pressure of pure solvent in Raoult's law?

The "pure solvent" is the solvent without solute, and the "solvent" (a clearer way is to simply say "solution") is the solvent with solute.

Raoult's law is (Physical Chemistry: A Molecular Approach, Ch. 25-2):

#\mathbf(a_1/chi_1 = P_1/(P_1^"*"chi_1) = gamma_1)#

where:

• #a_1# is the activity of the solvent. It is like a more accurate concentration.
• #gamma_1# is the activity coefficient of the solvent. When #gamma_1 = 1#, the solvent is pure.
• #chi_1# is the mole fraction of the solvent, and is equal to #(n_1)/(n_1 + n_2)#, where #n_2# is #"mol"#s of solute and #n_1# is #"mol"#s of solvent.
• #P_1# is the vapor pressure of the solvent (solution with solute).
• #P_1^"*"# is the vapor pressure of the pure solvent (solution without solute).

APPROACHING IMPURITY

Basically, the idea is if you add solute to a solvent, the mole fraction of solvent has decreased because a smaller percentage of the solution belongs to the solvent. So, what I mean is:

#chi_1 = n_1/(n_1 + n_2)#

becomes smaller as #n_2# (#"mol"#s of solute) increases. While #n_2# increases, the vapor pressure of the solvent in solution is called #P_1#, because the solution is impure (the solvent is not by itself).

Here's some example data using an equation from my book (Ch. 24) where I add solute to the solution. The solution starts with #"1 mol"# of solvent at #"120 bar"# pressure. Go rightwards on the curve to see the trend of adding more solute.

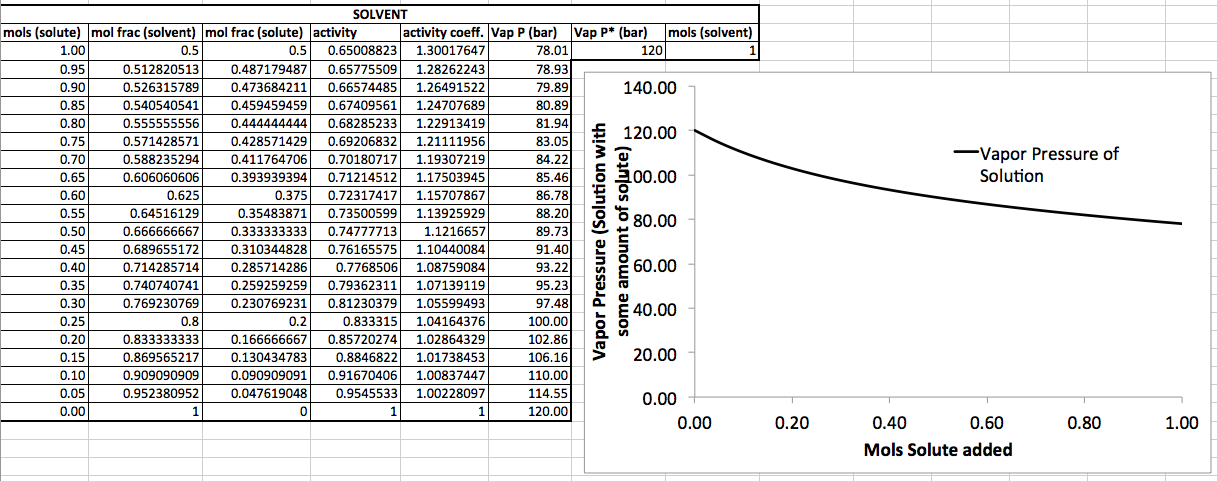
APPROACHING PURITY

Now, let's consider going the other way. That means you go leftwards on the curve.

If you "undissolve" the solute and then take solute out of the solution, it follows that you have decreased #n_2# and increased #chi_1# (downwards on the table).

Now, go all the way until #chi_1 = 1# (#n_2 = 0#), i.e. the solution is pure. It just so happens that as #chi_1 -> 1#, #a_1 -> chi_1# (meaning that #a_1 -> 1#).

Note the following relationship derived from Raoult's law:

#gamma_1 = a_1/chi_1#

Based on what we just said, it follows that #gamma_1 = 1#, which as I said earlier, tells you that the solution (and therefore solvent) is pure. Furthermore, as #chi_1->1#, since #a_1 -> 1#, #a_1/chi_1 -> 1# and #P_1->P_1^"*"chi_1#.

When #\mathbf(chi_1 = 1)#, #\mathbf(P_1 = P_1^"*")#.

That means the vapor pressure of the impure solvent and that of the pure solvent are equal. The only way that could be true is if there is no solute in solution, which makes sense because we've been removing the solute!

Thus, with no solute, the concentration of pure solvent to solution is #\mathbf(1:1)#, and #a_1 = chi_1 = gamma_1 = 1#.

So, the gist of it is that:

• #P_1# is the vapor pressure of the solvent for when #chi_1 < 1# and #a_1 < 1#. This means you have an impure solution (with solute in the solvent).
• #P_1^"*"# is the vapor pressure for the solvent when #chi_1 = a_1 = gamma_1 = 1#. Thus, #P_1 = P_1^"*"# for a pure solution (no solute in the solvent).