The following cell is operated as an electrolytic cell, using a current of 0.480 A . The volume of each solution is 100.0 mL. Electrolysis is stopped after 10.00 h , and the cell is allowed to function as a voltaic cell. What is #E_(cell)# at this point?

!! EXTREMELY LONG ANSWER !!

Let's start by calculating #E_"cell"^@#, the standard cell potential, for when the cell is allowed to function as a voltaic cell.

You know that you have

The balanced chemical equation that describes this redox reaction looks like this

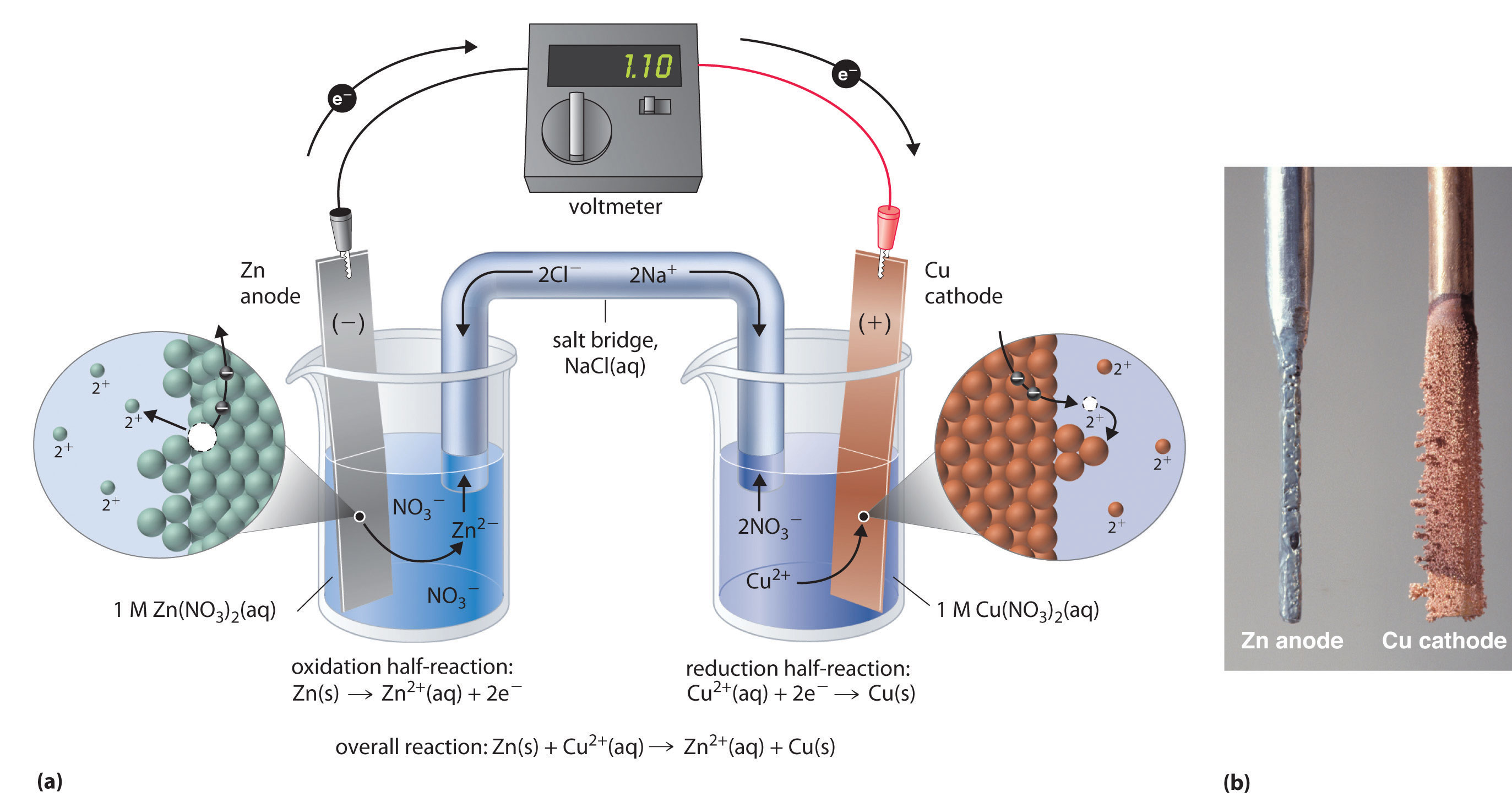
#"Zn"_ ((s)) + "Cu"_ ((aq))^(2+) -> "Zn"_ ((aq))^(2+) + "Cu"_ ((s))#

Write down the reduction half-reactions and the standard reduction potentials for zinc and for copper

#"Zn"_ ((aq))^(2+) + 2"e"^(-) -> "Zn"_ ((s))" "E_"red"^@ = - "0.763 V"#

#"Cu"_ ((aq))^(2+) + 2"e"^(-) -> "Cu"_ ((s))" "E_"red"^@ = + "0.337 V"#

http://www.chemeddl.org/services/moodle/media/QBank/GenChem/Tables/EStandardTable.htm

Now, notice that zinc is being oxidized to zinc cations at the anode, so reverse the reduction half-reaction for zinc to get the oxidation half-reaction

#"Zn"_ ((s)) -> "Zn"_ ((aq))^(2+) + 2"e"^(-)" "E_"oxi"^@ = - (-"0.763 V")#

You will have

#E_"oxi"^@ = - E_"red"^@#

#E_"oxi"^@ = - (-"0.763 V") = + "0.763 V"#

The standard cell potential is equal to

#E_"cell"^@ = E_"red"^@ + E_"oxi"^@#

In your case, this will be equal to

#E_"cell"^@ = +"0.337 V" + "0.763 V" = +"1.100 V"#

Keep this in mind because it will come in handy later.

Since you have #E_"cell"^@ > 0#, and consequently #DeltaG < 0#, you know that the forward reaction is spontaneous, i.e. the reaction takes place without the aid of an external power source.

In other words, when the cell is operating as a voltaic cell, you can expect the mass of the zinc electrode to decrease and the mass of the copper electrode to increase.

Now, you're operating this cell as an electrolytic cell. This means that you're using an external power source to force the reverse reaction to take place

#"Cu"_ ((s)) + "Zn"_ ((aq))^(2+) -> "Cu"_ ((aq))^(2+) + "Zn"_ ((s))#

In this case, copper metal is being oxidized to copper(II) cations, so you should expect the mass of the anode to decrease and the concentration of copper(II) cations to increase.

Zinc cations are being reduced to zinc metal, so you should expect the mass of the cathode to increase and the concentration of zinc cations to decrease.

So, you know that you're using a current of #"0.480 A"# for a duration of #"10.00 h"# to operate the cell as an electrolytic cell. Your goal here is to figure out how many moles of electrons are going to take part in the reaction.

As you know, you have

#"1 A" = "1 C"/"1 s"#

Your current delivers #"0.480 C"# of charge per second, which means that the total charge delivered in #"10.0 h"# will be equal to

#10.00 color(red)(cancel(color(black)("h"))) * (3600 color(red)(cancel(color(black)("s"))))/(1color(red)(cancel(color(black)("h")))) * "0.480 C"/(1color(red)(cancel(color(black)("s")))) = 1.728 * 10^4# #"C"#

Now, #1# mole of electrons carries a charge of #9.65 * 10^4# #"C"#. This implies that your current source delivers

#1.728 * 10^4 color(red)(cancel(color(black)("C"))) * "1 mole e"^(-)/(9.65 * 10^4color(red)(cancel(color(black)("C")))) = "0.1791 moles e"^(-)#

You have

#"Cu"_ ((s)) -> "Cu"_ ((aq))^(2+) + 2"e"^(-)#

Since #1# mole of copper metal is reduced to produce #1# mole of copper(II) cations and #2# moles of electrons, you can say that the reverse reaction produced

#0.1791 color(red)(cancel(color(black)("moles e"^(-)))) * "1 mole Cu"^(2+)/(2color(red)(cancel(color(black)("moles e"^(-))))) = "0.08955 moles Cu"^(2+)#

Similarly, you have

#"Zn"_ ((aq))^(2+) + 2"e"^(-) -> "Zn"_ ((s))#

This time, you consume #1# mole of zinc cations by using #2# moles of electrons, so you can say that the reverse reaction consumed

#0.1791 color(red)(cancel(color(black)("moles e"^(-)))) * "1 mole Zn"^(2+)/(2color(red)(cancel(color(black)("moles e"^(-))))) = "0.08955 moles Zn"^(2+)#

Use the molarities and the volumes of the two half-cells to calculate the initial number of moles of each cation

#100.0 color(red)(cancel(color(black)("mL"))) * "1.00 moles Cu"^(2+)/(10^3color(red)(cancel(color(black)("mL")))) = "0.100 moles Cu"^(2+)#

#100.0 color(red)(cancel(color(black)("mL"))) * "1.00 moles Zn"^(2+)/(10^3color(red)(cancel(color(black)("mL")))) = "0.100 moles Zn"^(2+)#

After you disconnect the power source, the resulting solutions will contain

#n_ ("Cu"^(2+)) = "0.100 moles" + "0.08955 moles" = "0.1896 moles Cu"^(2+)#

#n_ ("Zn"^(2+)) = "0.100 moles" - "0.08955 moles" = "0.01045 moles Cn"^(2+)#

Calculate the new concentrations of the two cations

#["Cu"^(2+)] = "0.1896 moles"/(100.0 * 10^3"L") = "1.896 M"#

#["Zn"^(2+)] = "0.01045 moles"/(100.0 * 10^3"L") = "0.1045 M"#

At this point, you allow the cell to function as a voltaic cell. You're no longer under standard conditions because the concentrations of the two solutions have changed, so you must calculate the cell potential using--you can assume that the temperature does not change, i.e. you're still working at #25^@"C"#

#E_"cell" = E_"cell"^@ - 0.0592/n * log(Q)#

Here

• #n# is the number of moles of electrons transferred in the redox reaction
• #Q# is the reaction quotient

Keep in mind that the cell is working as a voltaic cell again, so you have

#"Zn"_ ((s)) + "Cu"_ ((aq))^(2+) -> "Zn"_ ((aq))^(2+) + "Cu"_ ((s))#

The reaction quotient is equal to

#Q = (["Zn"^(2+)])/(["Cu"^(2+)])#

The number of moles of electrons transferred in the balanced redox reaction will be equal to #2# (the reduction and oxidation half-reactions show that this is the case), so you have

#E_"cell" = E_"cell"^@ - 0.0592/2 * log((["Zn"^(2+)])/(["Cu"^(2+)]))#

This will get you

#E_"cell" = +"1.100 V" - 0.0592/2 * log( (0.1045 color(red)(cancel(color(black)("M"))))/(1.896 color(red)(cancel(color(black)("M")))))#

#color(darkgreen)(ul(color(black)(E_"cell" = +"1.14 V")))#

The answer is rounded to three sig figs, the number of sig figs you have for the current.