What does the equation #(x+2)^2/4-(y+1)^2/16=1# tell me about its hyperbola?

Question

6747 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2015-01-15T03:46:27

Quite a lot!

Here, we have the standard hyperbolic equation.

#(x-h)^2/a^2-(y-k)^2/b^2=1#

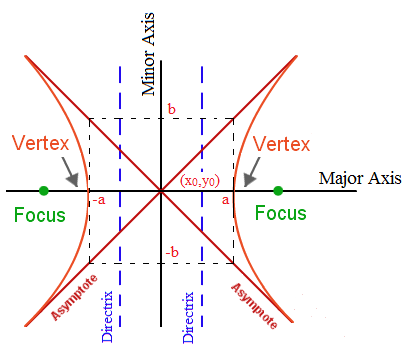
The center is at #(h,k)#

The semi-transverse axis is #a#

The semi-conjugate axis is #b#

The vertices of the graph are #(h+a, k)# and #(h-a, k)#

The foci of the graph are #(h+a*e, k)# and #(h-a*e, k)#

The directrices of the graph are #x = h + a/e# and #x = h - a/e#

Here is an image to help.

![http://formulas.tutorvista.com/math/http://hyperbola-formula.html](https://useruploads.socratic.org/YoUuy4cRSPCSUsRxTE0i_hyperbola.png)