Show that for a van der Waals gas, #((delC_V)/(delV))_T = 0#, where #C_V = ((delU)/(delT))_V#?
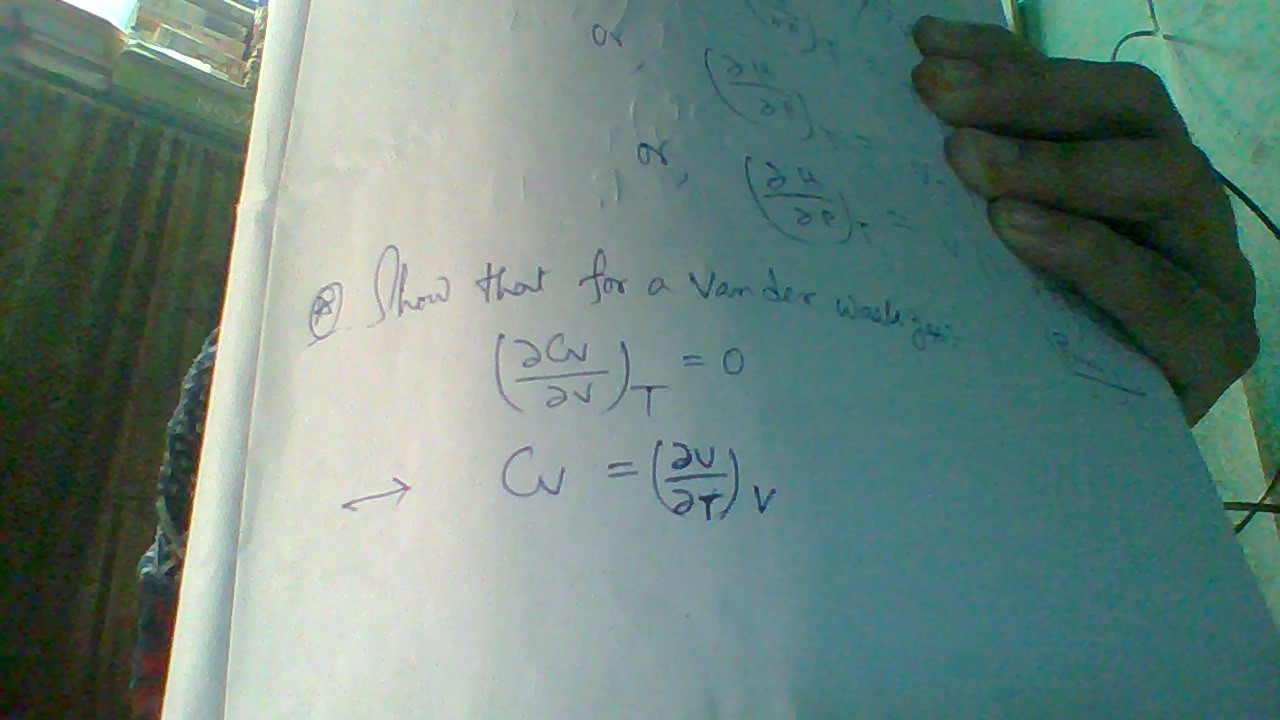
1 Answer
By definition, the constant-volume heat capacity was:
#C_V = ((delU)/(delT))_V# ,#" "" "bb((1))# where
#U# is the internal energy, and#T# and#V# are temperature and volume, respectively, as defined in the ideal gas law and other gas laws.
To show that for a van der Waals gas, the constant-volume heat capacity does not change due to a change in volume at a constant temperature, i.e.
#bb(P = (RT)/(barV - b) - a/(barV^2) = (nRT)/(V - nb) - (an^2)/(V^2))# ,#" "" "bb((2))# where
#barV = V/n# is the molar volume,#R# is the universal gas constant,#a# and#b# are the vdW constants for intermolecular forces of attraction and excluded volume (respectively), and#P# is the pressure.
This means we'll need to have an expression for
We can use the Maxwell relation for the internal energy in a closed system undergoing a reversible process:
#dU = TdS - PdV# ,
If we divide by
#((delU)/(delV))_T = T((delS)/(delV))_T - Pcancel(((delV)/(delV))_T)^(1)# #" "" "bb((3))#
This will be the main expression we'll work with. Note that
#dcolor(red)(A) = -Scolor(red)(dT) - Pcolor(red)(dV)#
Using the fact that the Helmholtz free energy is a state function (just like
#-((delS)/(delV))_T = -((delP)/(delT))_V#
Plugging into
#((delU)/(delV))_T = T((delP)/(delT))_V - P# #" "" "bb((4))# which is something we can relate back to the vdW equation of state, using
#((delP)/(delT))_V# . We're almost there.
Given that
#(del)/(delV)[((delU)/(delT))_V]_T = (del)/(delT)[((delU)/(delV))_T]_V#
or
#((del^2U)/(delVdelT))_(V,T) = ((del^2U)/(delTdelV))_(T,V)#
This will become relevant if we take the partial derivative of
#((delC_V)/(delV))_T = (del)/(delV)[C_V]_T#
#= (del)/(delV)[((delU)/(delT))_V]_T#
#= (del)/(delT)[((delU)/(delV))_T]_V# #" "" "bb((5))#
Now consider the right side of
#((delC_V)/(delV))_T#
#= (del)/(delT)[T((delP)/(delT))_V - P]_V#
#= (del)/(delT)[T((delP)/(delT))_V]_V - ((delP)/(delT))_V# #" "" "bb((6))#
Now, we should figure out what
#((delP)/(delT))_V = (del)/(delT)[(nRT)/(V - nb) - (an^2)/(V^2)]_V#
#= (nR)/(V - nb)#
Plugging this back into
#color(blue)(((delC_V)/(delV))_T) = (del)/(delT)[(nRT)/(V - nb)]_V - (nR)/(V - nb)#
#= (nR)/(V - nb) - (nR)/(V - nb)#
#= color(blue)(0)#

