Human body temperatures are normally distributed with a mean of 98.6 and a standard deviation of 0.72.? How do I Find the temperature that separates the top 7% from the bottom 93%?

1 Answer
Nallasivam V
Mar 3, 2017

Temprature that separates top 7% from the bottom 93% is #99.67#

Explanation:

Look at the diagram -
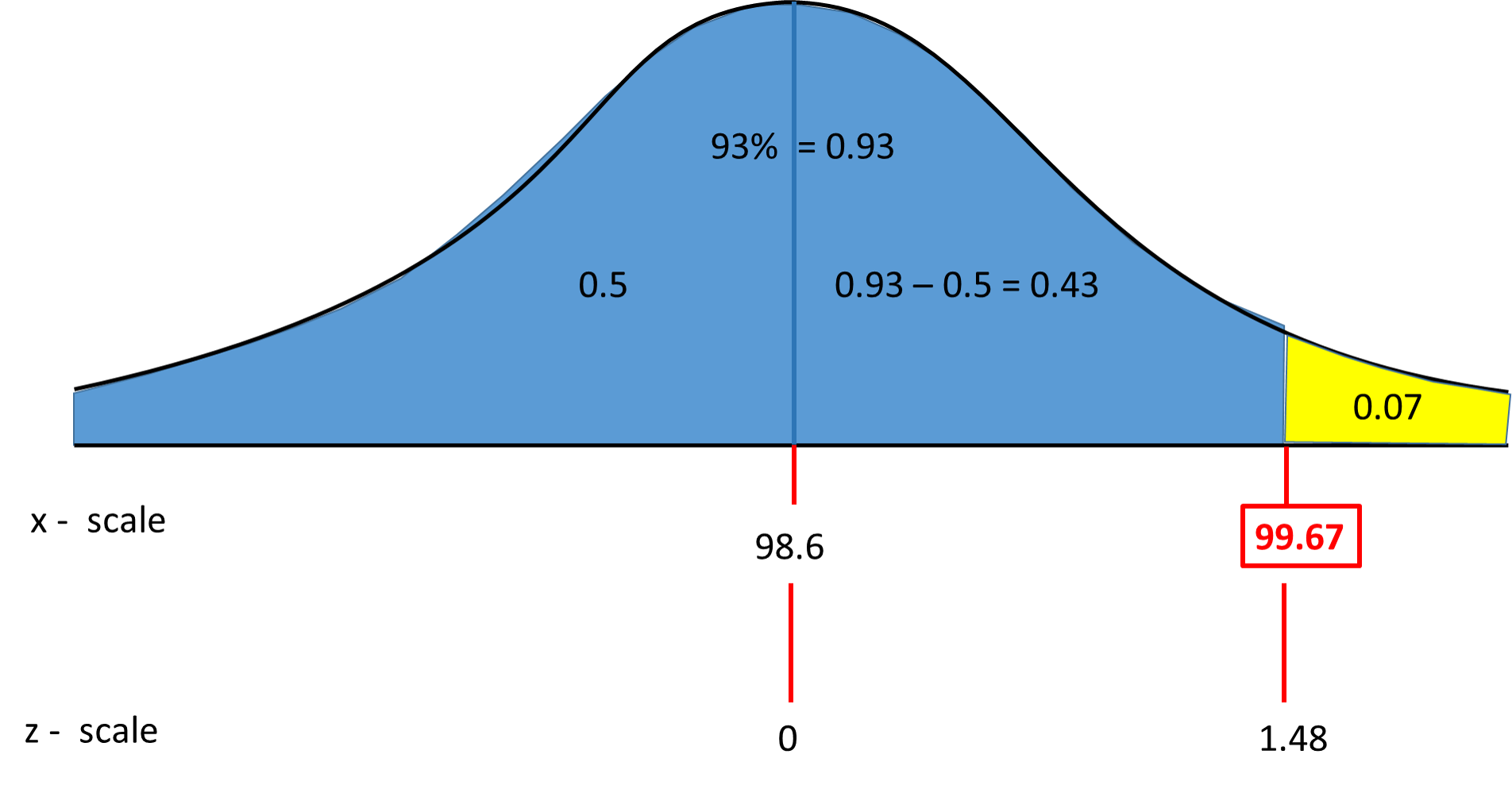
enter image source here

Find the probability area bound by 93% or 0.93.
0.5 lies to the left of the mean value.
#0.93 - 0.5= 0.43# lies to the right of mean.
Find the corresponding #z# value for #0.43#.
It is 1.48.

Use this value to determine the required #x# value.
You have to use the formula -

#z=(x-mu)/sigma#

Solve it for #x#

#x=z *sigma + mu#

Where -

#x = ?#
#z=1.48#
#sigma=0.72#
#mu = 98.6#

Plug in these values in the formula-

#x=(1.48*0.72)+98.6 = 1.07+98.6=99.67#

Temprature that separates top 7% from the bottom 93% is #99.67#

Related questions
See all questions in The Standard Normal Distribution
Impact of this question
10308 views around the world
You can reuse this answer
Creative Commons License