How do you solve #y = 2x^2 + 3x + 6#?

Question

4328 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2015-10-27T19:07:12

Assuming you mean:
What are the roots? (find x if y=0)
It does not cross the x-axis so has a complex number solution.
#c = 1/4(3 +-(sqrt(39))i)#

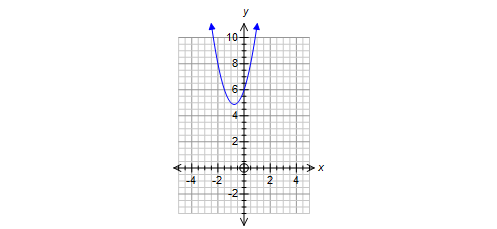
Tony B

Using standard form equations of:

#y =ax^2 + bx + c " "# and #" x = (-b+-sqrt(b^2 - 4ac))/(2a)#

Where #a=2; " " b = 3; " and " c =6# giving:

#x = (-3 +- sqrt( (3)^2 - 4(2)(6)))/(2(2) #

#x= (-3 +- sqrt( 9 - 48))/4 #

#x=-3/4 +- sqrt((-39))/4 #

But #-39 = 39 times (-1)#
and #sqrt(-1) = i#

giving: #x = 1/4(3 +-(sqrt(39))i)#