How do you graph #x^2 + (y + 2)^2 = 9#?

Question

3137 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2015-07-08T16:19:55

You convert the equation to standard form, determine the centre, vertices, and endpoints. Then you plot the graph.

#x^2 + (y+2)^2 = 9#

This is almost the standard form for the equation of a circle.

Let's modify it to read

#(x-0)^2 + (y+2)^2 = 9#

Now we see that it is a circle with centre at (#0,-2#) and radius #sqrt9 = 3#.

This means that, to find the vertices, you go 3 units up from the centre and 3 units down.

Thus, the vertices are at (#0,1#) and (#0,-5#).

To find the endpoints, you go 3 units left of the centre and 3 to the right.

Thus, the endpoints are at (#-3,-2#) and (#3,-2#).

Plot these points on a graph.

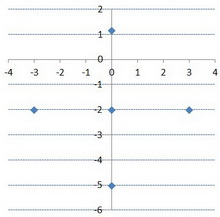
enter image source here

Now draw a smooth circle through these four points.

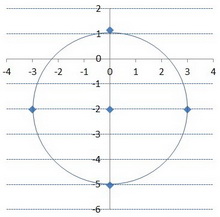
enter image source here

And you have your graph.