How do you find the slope that is perpendicular to the line #2x +3y = 5#?

Lines that are perpendicular will have negative reciprocal slopes. Meaning, if one line's slope is #m#, then a perpendicular line will have a slope of #-1/m#.

Why? A line's slope is equal to its rise over its run—also written as #m=[Delta y]/[Delta x]#. If we rotate that line 90° counterclockwise (making it perpendicular to its old self), the run (to the right) becomes a rise (up), and the rise (up) becomes a backwards run (to the left):

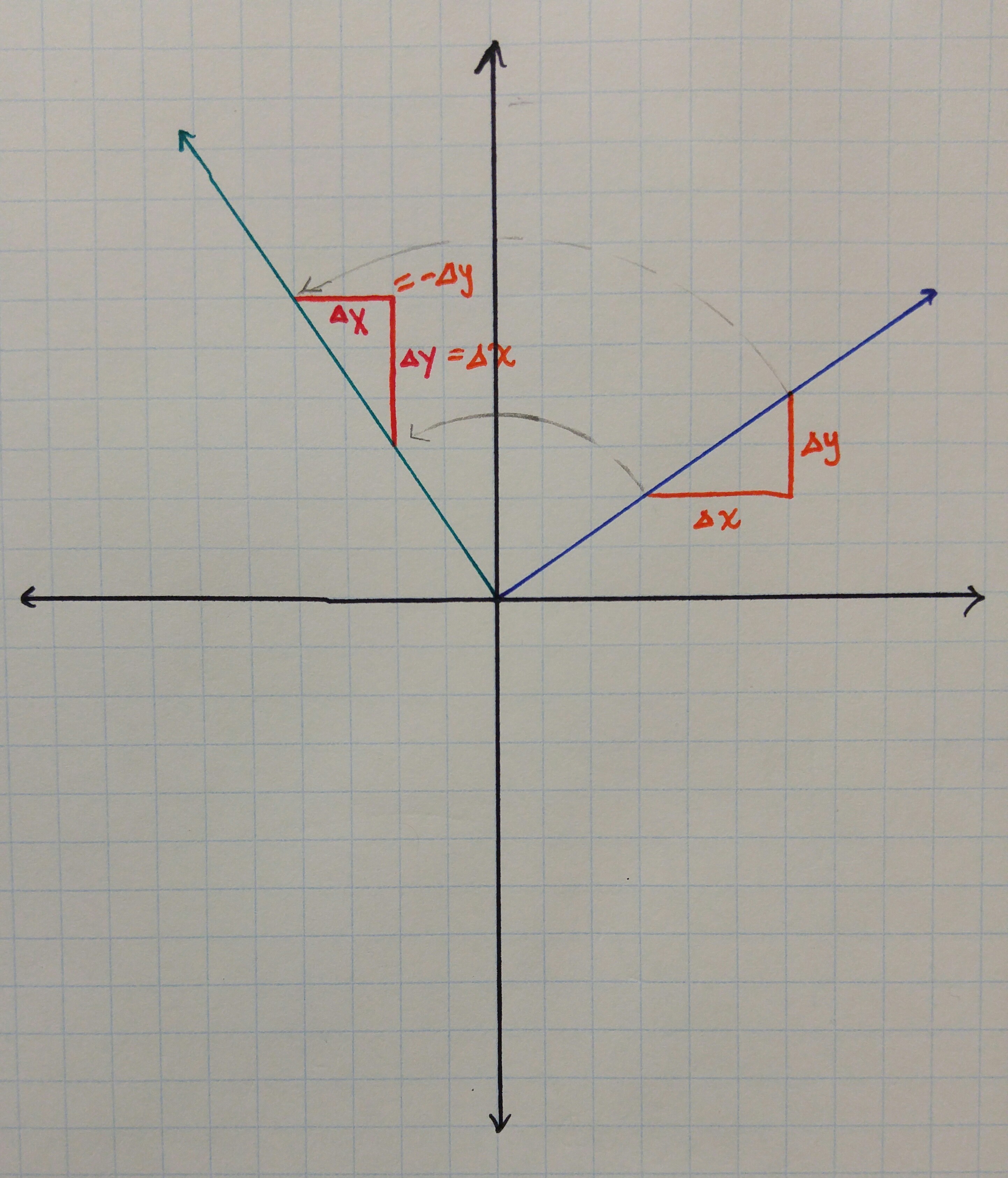
In math terms:

#Delta y_"new"=Delta x" "and" "Delta x_"new" = -Delta y#

thus

#m_"new"=(Delta y_"new")/(Delta x_"new")=(Delta x)/(-Delta y)=-(Delta x)/(Delta y)=-1/m#

(Note: if we rotate this new line another 90° (180° total from the beginning), this 3rd line will have a slope of #(-1)/(-1/m)#, which simplifies to #m#—the same slope of the first line, which is what we would expect.)

Okay, great—so what's the slope of #2x+3y=5#? If we rearrange this into slope-intercept form, we get

#y=-2/3 x+5/3#,

meaning that for every step of "2 down", we have a step of "3 right".

The negative reciprocal of the slope #m=-2/3# is

#m_"new"=-1/m=(-1)/(- 2/3)=3/2#,

meaning that, for a perpendicular line, a step of "3 up" comes with a step of "2 right".