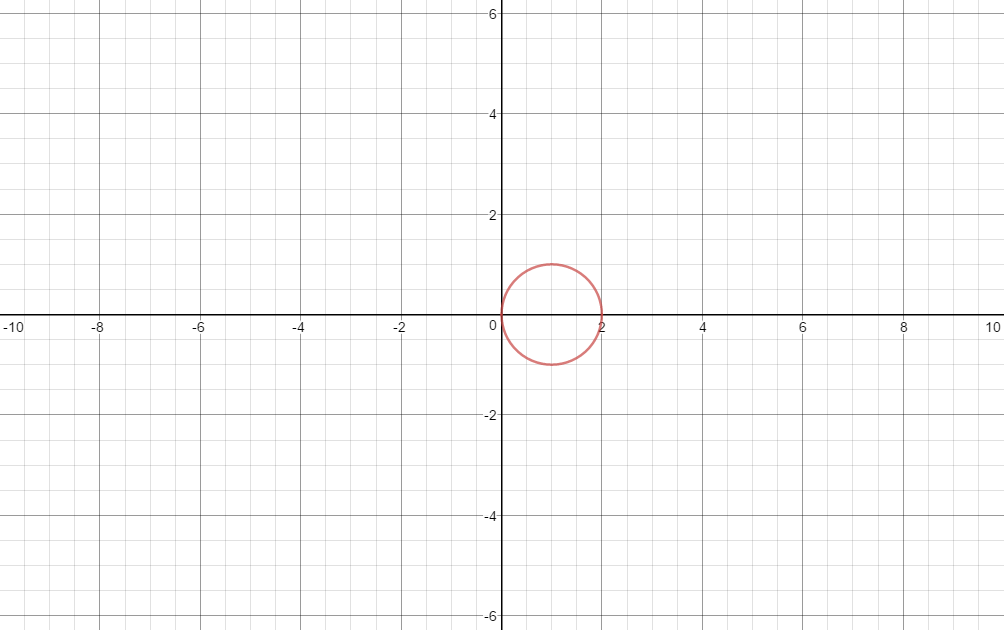
How do you find the rectangular equation for #r=2costheta#?

Question

2479 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-11-07T23:48:56

Please see the explanation for steps leading to the rectangular equation.

Multiply both sides by r:

#r^2 = 2rcos(theta)#

Substitute #(x^2 + y^2)# for #r^2# and x for #rcos(theta)#:

#x^2 + y^2 = 2x#

Add #h^2 - 2x# to both sides:

#x^2 - 2x + h^2 + y^2 = h^2#

Use the right side of the pattern #(x - h)^2 = x^2 - 2hx + h^2# equal to the first 3 terms to find the value of h:

#x^2 - 2hx + h^2 = x^2 - 2x + h^2#

#-2hx = -2x#

#h = 1#

Substitute the left side of the pattern with h = 1 and the right side becomes #1^2#

#(x - 1)^2 + y^2 = 1^2#

It is a circle with center of #(1, 0)# and radius of 1:

Here is a graph

enter image source here