How do you convert #r = 16 / 3 -5 cos theta# into cartesian form?

Question

3120 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-01-30T18:01:20

Please see the explanation.

Here is the graph of #r = 16/3 - 5cos(theta)#

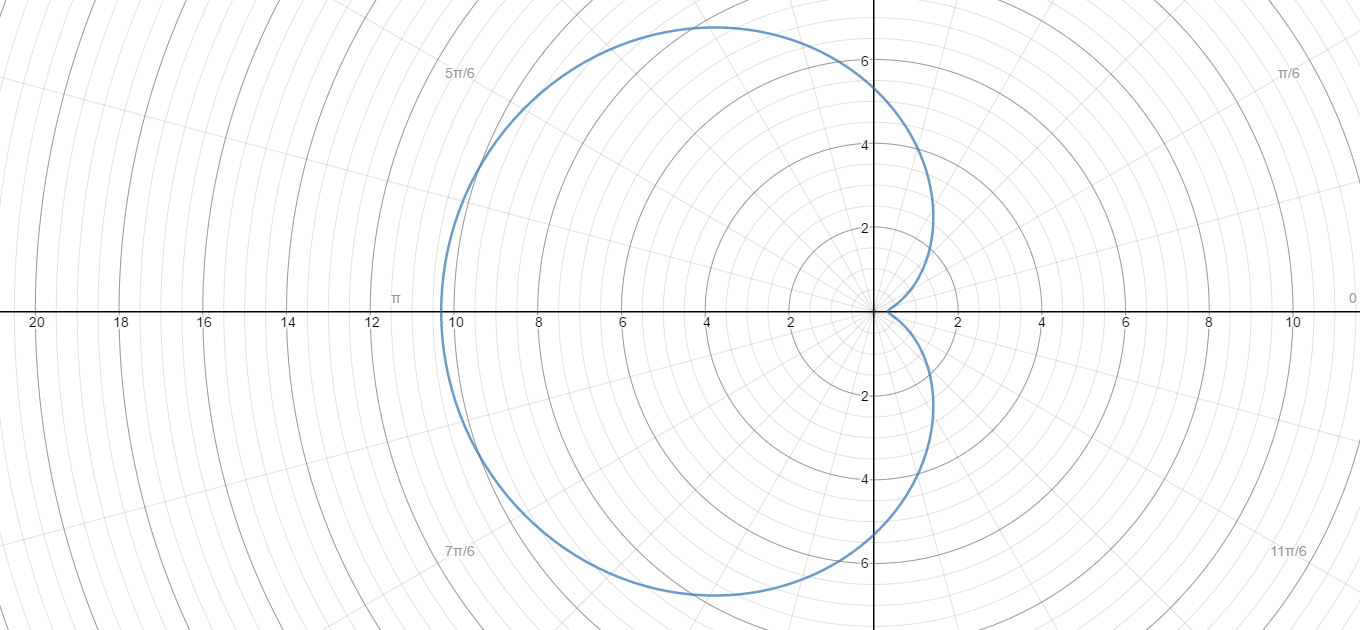
Desmos.com

Multiply both sides of the equation by r:

#r^2 = 16/3r-5rcos(theta)#

Subsitute #x^2+y^2" for "r^2#:

#x^2+y^2 = 16/3r-5rcos(theta)#

Substitute #sqrt(x^2+y^2)# for r:

#x^2+y^2 = 16/3sqrt(x^2+y^2)-5rcos(theta)#

Substitute x for #rcos(theta)#

#(x^2+y^2) = 16/3sqrt(x^2+y^2)-5x#

Here is a graph of the converted equation:

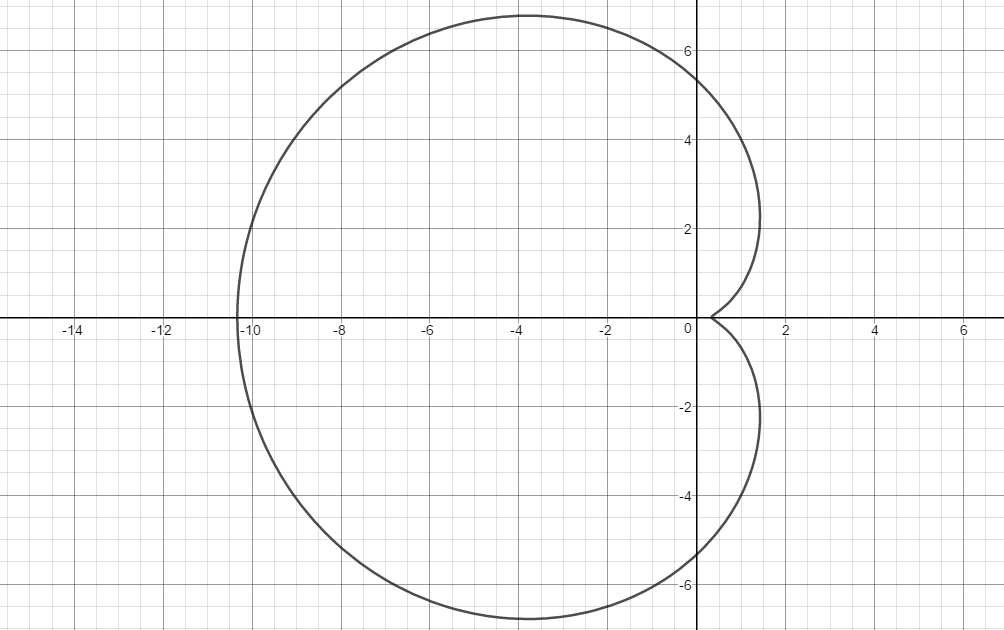
Desmos.com

Please observe that the graphs are identical.