How are more complex red-ox reactions balanced? The kind of reactions which have 3 or more reactions and multiple products?Also what do we do when more that one species is undergoing oxidation/reduction?

I got:

#2"MnO"_4^(-)(aq) + 6"H"^(+)(aq) + 5"H"_2"O"_2(aq) -> 2"Mn"^(2+)(aq) + 5"O"_2(g) + 8"H"_2"O"(l)#

or

#2"KMnO"_4(aq) + 3"H"_2"SO"_4(aq) + 5"H"_2"O"_2(aq) -> 2"MnSO"_4(aq) + "K"_2"SO"_4(aq) + 5"O"_2(g) + 8"H"_2"O"(l)#

Oxygen in #"H"_2"O"_2# was oxidized, and manganese in #"MnO"_4^(-)# was reduced.

DISCLAIMER: LONG ANSWER!

Normally, we would start with a half-reaction and work towards the final balanced reaction.

I don't know how you got the unbalanced semi-final result, but I guess:

1. We'll work backwards to find the half-reactions.
2. We'll go back forwards to balance the reaction itself in acidic conditions.

ROUGHLY IDENTIFYING WHICH TWO REACTIONS ARE PRESENT

#"KMnO"_4(aq) + "H"_2"SO"_4(aq) + "H"_2"O"_2(aq) -> "MnSO"_4(aq) + "K"_2"SO"_4(aq) + "O"_2(g) + "H"_2"O"(l)#

If you focus on what's similar on either side of the reaction, you should see that sulfate, potassium, and manganese stand out.

#color(highlight)(overbrace("KMnO"_4(aq)) + overbrace("H"_2"SO"_4(aq))) + color(red)("H"_2"O"_2(aq)) -> color(highlight)(overbrace("MnSO"_4(aq)) + overbrace("K"_2"SO"_4(aq))) + color(red)("O"_2(g) + "H"_2"O"(l))#

This tells me that these compounds have been participating in the same reaction.

REMOVING SPECTATOR IONS

Notice how #"K"^(+)# would be a spectator ion, because it is an alkali metal, which, from the solubility rules you should know, renders the resultant ionic compound quite soluble.

#"MnSO"_4# is very soluble in water, so #"SO"_4^(2-)# is another spectator ion. Also, #"H"_2"SO"_4# was there because the solution was under acidic conditions.

We can simply call that #2"H"^(+)#, because enough of the sulfuric acid was added to make the conditions reducing enough (we'll look at why soon).

Thus, we have the following unbalanced ionic equation to decompose:

#color(red)(mathbf("MnO"_4^(-)(aq) + 2"H"^(+)(aq) + "H"_2"O"_2(aq) -> "Mn"^(2+)(aq) + "O"_2(g) + "H"_2"O"(l)))#

IDENTIFYING THE HALF-REACTIONS

Note that some of these species should be removed to give the true starting half-reactions, as they were added in the steps required to balance these reactions in the first place.

As we identified earlier, the previously bracketed/unbracketed compounds can be separated into their own separate half reactions:

#"MnO"_4^(-)(aq) + stackrel("shouldn't be here")overbrace(cancel(2"H"^(+)(aq))) -> "Mn"^(2+)(aq)# (1)

#"H"_2"O"_2(aq) -> "O"_2(g) + stackrel("shouldn't be here")overbrace(cancel("H"_2"O"(l)))# (2)

These are much easier to balance, right? Now, which one is reduction and which one is oxidation? Let's look at how to distinguish them.

BALANCING THE HALF-REACTIONS

(1)

A nice starting half-reaction containing only manganese species derived from (1) is:

#\mathbf("MnO"_4^(-) -> "Mn"^(2+))#

According to this Pourbaix diagram (remember this?), this can occur, let's say, at #"pH" = 2#, and involves at least #"0.6 V"#.

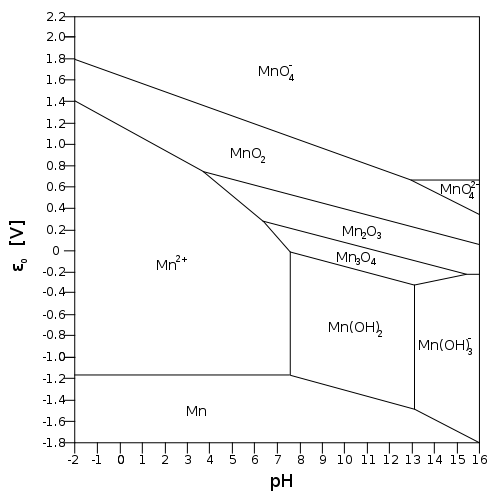
Recall that on a Pourbaix diagram, going downwards requires adding a negative voltage. Also, to minimize the number of reaction intermediates, we can do this at #"pH" = 2#.

That's why "enough" #"H"_2"SO"_4# was there: to help reach that acidic #\mathbf("pH")# and maximize reaction yield.

Now, balancing this, we "add" water to balance the oxygens:

#"MnO"_4^(-)(aq) -> "Mn"^(2+)(aq) + color(red)(4"H"_2"O"(l))#

Then we add protons to balance the hydrogens in acidic conditions:

#"MnO"_4^(-)(aq) + color(red)(8"H"^(+)(aq)) -> "Mn"^(2+)(aq) + 4"H"_2"O"(l)#

Finally, add electrons to balance the charge.

#color(blue)(stackrel(color(red)(+7))("Mn")stackrel(color(red)(-2))("O"_4^(-))(aq) + 8stackrel(color(red)(+1))("H"^(+))(aq) + 5e^(-) -> stackrel(color(red)(+2))("Mn"^(2+))(aq) + 4stackrel(color(red)(+1))("H")_2stackrel(color(red)(-2))("O")(l))#

Indeed, this is a real half-reaction, and it involves #pm"1.51 V"#.

From examining the oxidation states, we see that manganese was reduced as #stackrel(+7)("Mn") -> "Mn"^(2+)# (#"MnO"_4^(-)# was the oxidizing agent).

Thus, this is the reduction half-reaction.

(2)

The other one is more tricky, as there are two possibilities. You could go to either water, or oxygen gas. However, since you assume that oxygen gas is produced...

I found a half-reaction here that starts as:

#\mathbf("H"_2"O"_2(aq) -> "O"_2(g))#

To balance this, we repeat the process we did before for the reduction half-reaction, except we don't need to "add" water because the oxygens are balanced.

#"H"_2"O"_2(aq) -> "O"_2(g) + color(red)(2"H"^(+)(aq))#

Since the charge is now unbalanced, let's re-balance it.

#color(blue)(stackrel(color(red)(+1))("H")_2stackrel(color(red)(-1))("O")_2(aq) -> stackrel(color(red)(0))("O")_2(g) + 2stackrel(color(red)(+1))("H"^(+))(aq) + 2e^(-))#

And from here you can see that oxygen was oxidized, as #stackrel(-1)("O") -> stackrel(0)("O")# (#"H"_2"O"_2# was the reducing agent).

Thus, this is the oxidation half-reaction.

COMBINING THE HALF-REACTIONS BACK TOGETHER

Finally, we can put these two half-reactions together and get the result by making sure the electrons cancel out (as they were merely an accounting scheme):

#2("MnO"_4^(-)(aq) + 8"H"^(+)(aq) + cancel(5e^(-)) -> "Mn"^(2+)(aq) + 4"H"_2"O"(l))#
#5("H"_2"O"_2(aq) -> "O"_2(g) + 2"H"^(+)(aq) + cancel(2e^(-)))#
#"-----------------------------------------------------------"#

#\mathbf(color(blue)(2"MnO"_4^(-)(aq) + 6"H"^(+)(aq) + 5"H"_2"O"_2(aq) -> 2"Mn"^(2+)(aq) + 5"O"_2(g) + 8"H"_2"O"(l)))#

That's good enough.

But... if we add back all the spectator ions and totally soluble ionic compounds, we would get:

#color(blue)(2"KMnO"_4(aq) + 3"H"_2"SO"_4(aq) + 5"H"_2"O"_2(aq) -> 2"MnSO"_4(aq) + "K"_2"SO"_4(aq) + 5"O"_2(g) + 8"H"_2"O"(l))#

https://socratic.org/questions/how-to-balance-this-equation-using-oxidation-number-method-kmno4-h2so4-h2o2-mnso