Are there any geometric isomers of the stable octahedral complex #[Co(NH_3)_3(NO_2)_3]#? If there are, how many?

The #Co(III)# has two geometric isomers available: facial, 3 ligands occupy the face of an octahedron; meridional, where 2 of the ligands are trans, and the third is cis.

You could use the Bailar method, gone through in detail here, to determine how many isomers there are, as it is more generalized for all octahedral complexes.

However, since we have a #Ma_3b_3# complex where #a = "NH"_3# and #b = "NO"_2^(-)#, and neither is polydentate, this is one of those special cases where we have only two possible isomers: fac and mer.

THE FAC ISOMER

The fac (facial) isomer has the three identical ligands aligned such that there is a #C_3# axis through the three ligands. That is, it can be drawn in a Newman projection with a front-rear bond length of #\mathbf(0)#.

What I had just said can be drawn as follows:

So you can see that if you rotate the molecule about that #C_3# axis by #120^@#, you get the same molecule back.

Furthermore, you can find a mirror plane that coincides with an #a# and #b# ligand trans to each other, and bisects two cis #a# or #b# ligands, which tells you that the isomer is not chiral.

Therefore, the fac isomer is NOT an enantiomeric isomer and is the only fac isomer there is.

THE MER ISOMER

The mer (meridian) isomer has three #a# and one #b# equatorial ligands, and two #b# axial ligands. The ligand arrangement resembles the actual meridian, relative to the horizon.

In essence, I switched the top-axial #a# ligand and the rear-equatorial #b# ligand.

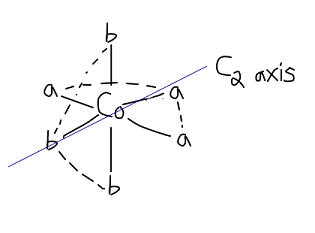
So you can see that if you rotate the molecule about that #C_2# axis by #180^@#, you get the same molecule back.

Furthermore, you can find a mirror plane that is coplanar with the #b# ligands and bisects the #a#-ligand plane, which tells you that the isomer is not chiral.

Therefore, the mer isomer is NOT an enantiomeric isomer and is the only mer isomer there is.

CHECKING THE RESULT

In fact, if you look at the following table, the #Ma_3b_3# isomer we have indeed has only two stereoisomers, neither of which are enantiomers.

Since a geometric isomer has the same connectivity but different spatial orientations, these stereoisomers, which agree with that definition, are also geometric isomers!

Thus, we have all geometric isomers identified.