A flask containing 155 #cm^3# of hydrogen was collected under a pressure of 22.5 #kPa#. What pressure would have been required for the volume of the gas to have been 90.0 #cm^3#, assuming the same temperature?

Before doing any calculations, try to predict what you expect the required pressure to be relative to the given pressure of #"22.5 kPa"#.

As you know, pressure and volume have an inverse relationship when temperature and number of moles are kept constant - this is known as Boyle's Law.

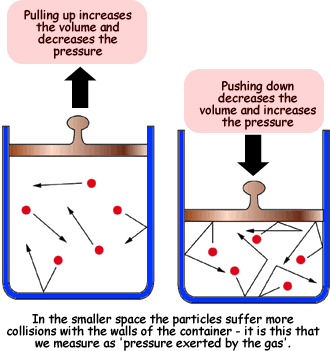
Simply put, when volume increases, the pressure exerted by the gas molecules decreases. Likewise, when volume decreases, the pressure exerted by the gas molecules increases.

In your case, you want to know what pressure would have resulted in a smaller volume of hydrogen gas. Well, in order for the volume to be smaller, you'd need to have a higher pressure.

You can thus expect the required pressure to be lower than the given #"22.5 kPa"#.

Mathematically, Boyle's Law is expressed like this

#color(blue)(P_1V_1 = P_2V_2)" "#, where

#P_1#, #V_1# - the pressure and volume of the gas at an initial state
#P_2#, #V_2# - the pressure and volume of the gas at a final state

Rearrange the equation to solve for #P_2#

#P_2 = V_1/V_2 * P_1#

Plug in your values to get

#P_2 = (155 color(red)(cancel(color(black)("cm"^3))))/(90.0color(red)(cancel(color(black)("cm"^3)))) * "22.5 kPa" = color(green)("38.8 kPa")#

The answer is rounded to three sig figs.

So, the same number of moles of hydrogen gas kept at the same temperature will occupy a volume of #"155 cm"^3# at a pressure of #"22.5 kPa"# and a volume of #"90.0 cm"^3# at a pressure of #"38.8 kPa"#.