Question #6a5d5

The raising of the boiling point is one of the colligative properties of solutions.

Being a colligative property, the effect is independent of the identity of the solute, but only the amount of solute in solution.

The equation for boiling point elevation is

#DeltaT_b = imK_b#

where

• #DeltaT_b# is the change in boiling point (a positive quantity) of the solution

• #i# is the van't Hoff factor, which is essentially the number of dissolved ions per unit of solvent (assuming #"HCl"# is the solute, this value is #2#, because there is #1# #"H"^+# ion and #1# #"Cl"^-# ion per unit of #"HCl"#)

• #m# is the molality of the solution;

#"molality" = "mol solute"/"kg solvent"#

If we can figure out these two quantities, we can figure out the solution's molality.

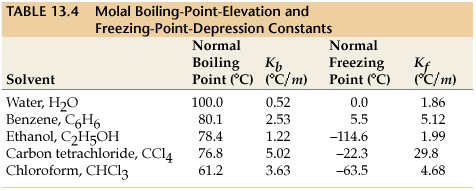
• #K_b# is the molal boiling point elevation constant for the solvent (assumed to be benzene). We can find this value from a list online:

From this, we can see that #K_b# for benzene is #color(red)(2.53# #color(red)(""^"o""C/"m#.

Plugging in the known values for the situation, we have

#DeltaT_b = (2)m(color(red)(2.53)color(white)(l)color(red)(""^"o""C/"m))#

#DeltaT_b = (5.06color(white)(l)^"o""C/"m)*m#

As long as we know the molal concentration #m#, we can find the increase in boiling point of this solution.

Let's say there is #1.00# #"mol HCl"# dissolved in #2.00# #"kg benzene"#.

The molality would be

#"molality" = (1.00color(white)(l)"mol HCl")/(2.00color(white)(l)"kg benzene") = color(green)(0.500m#

Plugging this into the equation, we can find the boiling point elevation:

#DeltaT_b = (5.06color(white)(l)^"o""C/"cancel(m))(color(green)(0.500)cancel(color(green)(m))) = 2.53# #""^"o""C"#

This quantity represents by how much the boiling point of the solvent (benzene) increases; to find the new boiling point of this solution, we simply add this to the normal boiling point of benzene (according to the above figure, it is #80.1# #""^"o""C"#):

#"new b.p." = 80.1^"o""C" + 2.53^"o""C" = color(blue)(82.6# #color(blue)(""^"o""C"#

rounded to one decimal place.