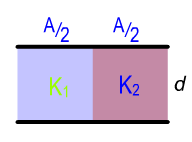
We have a case of a parallel-plate capacitor that has plate area A with a plate separation of d filled with dielectric strontium titanate up to half depth, similar to the figure as shown above. The remaining depth is empty and therefore has air as dielectric. It is clear that both dielectrics cover half the areaA/2 of the parallel plates.
Capacitance C=(kepsilon_0A)/d ......(1)
where k is relative permittivity of dieletric material, and epsilon_0 is permittivity of free space =8.854xx10^-12Fm^-1
k=1 for free space, k>1 for all media and kapprox1 for air.
Both dielectrics k_1 ("air")and k_2("strontium titanate") experience the same potential difference as both are connected to the same parallel plates on either sides. This is exactly the same situation as two capacitors connected in parallel where
Total capacitance C_"total"=C_"air"+C_"dielectric" .....(2)
Using (1) and information given above
C_"total"=(epsilon_0A/2)/d+(kepsilon_0A/2)/d
=>C_"total"=(epsilon_0A)/(2d)(1+k)
Taking the relative permittivity of strontium titanate as 310 we get
C_"total"=(311epsilon_0A)/(2d)
Inserting given values in SI units we get
C_"total"=(311xx8.854xx10^-12xx200/10^4)/(2xx4/10^3)
=>C_"total"=6.88xx10^-9F, rounded to two decimal places.
