Science

Math

Humanities

... and beyond

Socratic Meta
Featured Answers

Topics

Analyzing Polar Equations for Conic Sections

Key Questions

How do I find the directrix and focus of a parabola?

There are two basic kinds of parabola(as it is convenient for me to say)

Type 1:
The parabola lying on, or parallel to the $x -$ $x-$ axis

This parabola is of the form ${(y - y_{v})}_{v}^{2} = 4 a (x - x_{v})$ $(y-y_v)^2=4a(x-x_v)$

Where,
- focus is $(a + x_{v}, y_{v})$ $(a+x_v,y_v)$
- directrix is the line $x = x_{v} - a$ $x=x_v-a$
- Vertex is $(x_{v}, y_{v})$ $(x_v,y_v)$

Type 2:
The parabola lying on, or parallel to the $y -$ $y-$ axis

This parabola is of the form ${(x - x_{v})}_{v}^{2} = 4 a (y - y_{v})$ $(x-x_v)^2=4a(y-y_v)$

Where,
- focus is $(x_{v}, a + y_{v})$ $(x_v,a+y_v)$
- directrix is the line $y = y_{v} - a$ $y=y_v-a$
- Vertex is $(x_{v}, y_{v})$ $(x_v,y_v)$

Antoine · 1 · Apr 18 2015
How do I find the directrix of a hyperbola?

Answer:

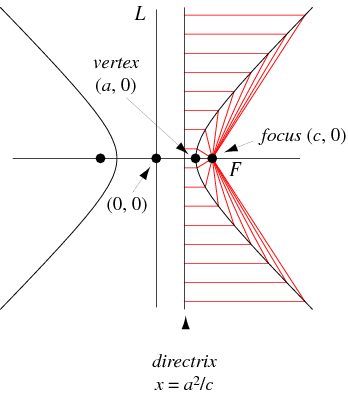
The directrix is the vertical line $x = \frac{a^{2}}{c}$ $x=(a^2)/c$ .

Explanation:

For a hyperbola $\frac{{(x - h)}^{2}}{a^{2}} - \frac{{(y - k)}^{2}}{b^{2}} = 1$ $(x-h)^2/a^2-(y-k)^2/b^2=1$ ,

where $a^{2} + b^{2} = c^{2}$ $a^2+b^2=c^2$ ,

the directrix is the line $x = \frac{a^{2}}{c}$ $x=a^2/c$ .

mason m · 14 · Jan 1 2016

Questions

Polar Equations of Conic Sections

View all chapters

Prev