How do you identity if the equation #2x^2+12x+18-y^2=3(2-y^2)+4y# is a parabola, circle, ellipse, or hyperbola and how do you graph it?

From the reference Conic Sections - General Cartesian Form , the form is:

#Ax^2 + Bxy + Cy^2 + Dx + Ey + F = 0" [1]"#

Rewrite the given equation to match this form:

#2x^2 + 0xy + 2y^2 + 12x - 4y + 12 = 0" [2]"#

#A = 2, B = 0, and C = 2#

The discriminant is:

#B^2 - 4AC = -16#

The conditions say that, when the discriminant is less than zero, then the equation represents and ellipse but, when #A = C#, the equation is a special case of an ellipse; a circle. Therefore, this equation is a circle and it must fit the standard Cartesian form for a circle:

#(x - h)^2 + (y - k)^2 = r^2" [3]"#

where x and y correspond to any point #(x, y)# on the circle, h and k correspond to the center point #(h, k)#, and r is the radius.

Expand the squares of equation [3]:

#x^2 - 2hx + h^2 + y^2 - 2ky + k^2 = r^2" [4]"#

To make equation [2] look more like equation [4] we divide both sides by 2 and move the constant term to the right side:

#x^2 + y^2 + 6x - 2y = -6" [5]"#

Add #h^2 and k^2# to both sides of the equation:

#x^2 + 6x + h^2 + y^2 - 2y + k^2 = h^2 + k^2 -6" [6]"#

We can find the value of h is we set the second term in equation [4] equal to the second term in equation [6] equal:

#-2hx = 6x#

#h = -3#

We can do the same for k with the fifth terms in equations [4] and [6]:

#-2kx = -2x#

#k = 1#

Now that we know the values of h and k, we can substitute the squares into equation [6] and compute the values of #h^2 and k^2# on the right:

#(x - -3)^2 + (y - 1)^2 = (-3)^2 + 1^2 -6 = 9 + 1 - 6 = 4" [7]"#

Write the right side as a square:

#(x - -3)^2 + (y - 1)^2 = 2^2" [8]"#

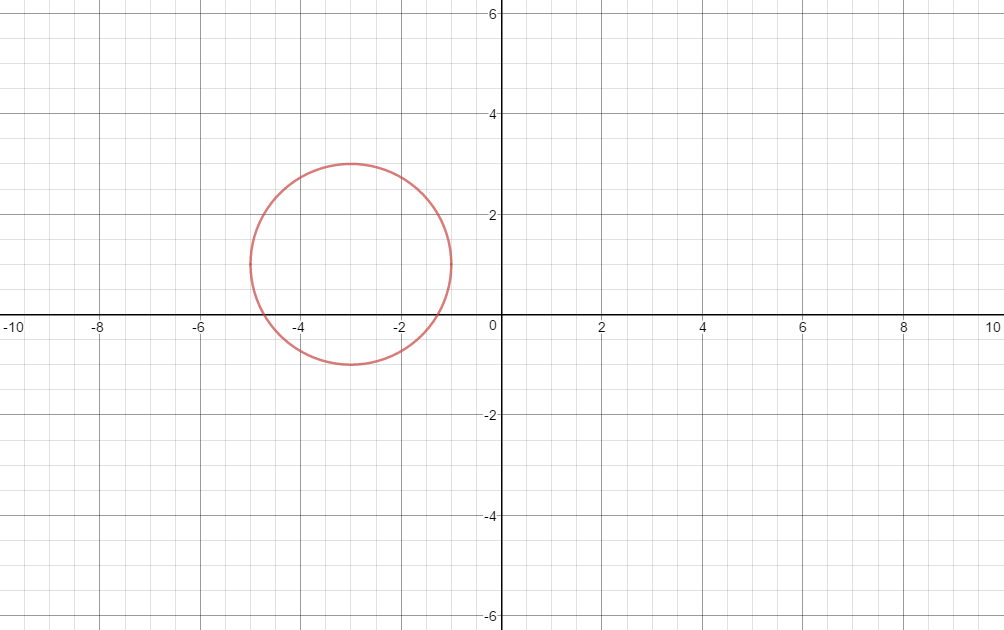
To graph equation [8], set your compass to a radius of 2, make the center point #(-3,1)# and draw a circle.

Here is a graph: