What is the equation of the line tangent to # f(x)=ln(x^2+3)/x # at # x=-1 #?

Question

2366 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-11-24T04:54:03

#y=(1/2-ln 4)x+1/2-ln 16#

the given function
#f(x)=(ln (x^2+3))/x#
at #x=-1#

Solve for the point first

#f(-1)=(ln ((-1)^2+3))/(-1)#

#f(-1)=y=-ln 4#

the point of tangency #(-1, -ln 4)#

Solve for the slope #m=f' (-1)#

take the first derivative

#f' (x)=(x*d/dx(ln(x^2+3))-ln(x^2+3)*d/dx(x))/(x^2)#

#f' (x)=(x*1/(x^2+3)*(2x+0)-ln(x^2+3)(1))/(x^2)#

Compute slope #f' (-1)#

#f' (-1)=((-1)(1/((-1)^2+3))(2(-1)+0)-ln((-1)^2+3)(1))/((-1)^2)#

#f' (-1)=1/2-ln 4#

Write the equation of the line using Point-Slope Form

#y-(-ln 4)=(1/2-ln 4)*(x-(-1))#

#y=(1/2-ln 4)*x+1/2-ln 16#

Kindly see the graph for better explanation

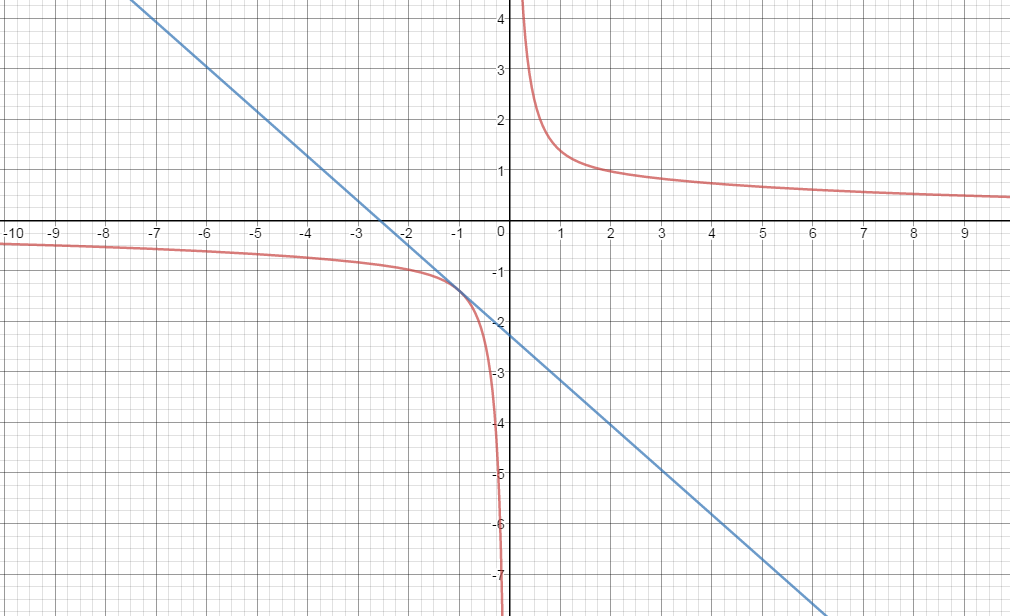
desmos.com

God bless....I hope the explanation is useful.