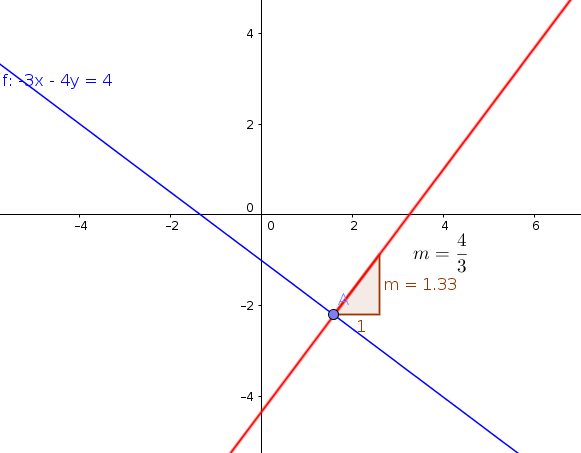
How do you find the slope that is perpendicular to the line #-6x -8y = 8#?

2 Answers
Deepak G.
Aug 4, 2016

#=4/3#

Explanation:

#y=mx+c# where #m# is the slope
#-6x-8y=8#
or
#8y=-6x-8#
or
#y=-6x/8-8/8#
or
#y=-3x/4-1#
So the slope is #m=-3/4# of the above lineSo the slope of the line that is perpendicular to the above line#=m1=-1/m=4/3#

ali ergin
Aug 4, 2016

#m_2=4/3#

Explanation:

#-6x-8y=8#

#"rearrange the equation -6x-8y=8 as :"#

#8y=-6x-8#

#y=-6/8x-8/8#

#y=color(red)(-3/4)x-1#

#"slope of the line is "-3/4#

#"the lines are perpendicular if product of their slopes are equal to -1"#

#m_1*m_2=-1#

#-3/4*m_2=-1#

#m_2=4/3#

enter image source here

Related questions
See all questions in Equations of Perpendicular Lines
Impact of this question
3996 views around the world
You can reuse this answer
Creative Commons License