How do you solve a triangle given <A=84.2°, <B=20.7°, B=17.2?

1 Answer
Noah G · EZ as pi
Apr 24, 2016

The following diagram represents your problem

Explanation:

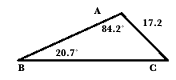
enter image source here

Step 1:

Since we know an angle opposite a known side, we can use the Sine Rule to solve for side a.

#sinA/a = sinB/b = sinC/c#

#sin84.2/a = sin20.7/17.2 = sinC/c#

#a = (17.2 xx sin84.2)/sin20.7#

#a = 48.4#

Step 2:

We could have done this at first, but let's find angle C. We know that the sum of the three angles in a triangle always equals 180. Therefore, we can set up the following equation:

#C + 20.7 + 84.2 = 180#

#C = 180 - 20.7 - 84.2#

#C = 75.1˚#

Step 3:

We must now substitute the value of angle C to find side C.

#SinB/b = sinC/c#

#sin20.7/17.2 = sin75.1/c#

#(17.2 xx sin75.1)/sin20.7 = c#

#47.0 = c#

Summary:

Your triangle has the following dimensions:

#/_A = 84.2˚#
#/_B = 20.7˚#
#/_C = 75.1˚#

#a = 48.4# units
#b = 17.2# units
#c = 47.0# units

Hopefully this helps!

Related questions
See all questions in The Law of Cosines
Impact of this question
1627 views around the world
You can reuse this answer
Creative Commons License