How do you find the axis of symmetry and vertex point of the function: #y=16x-4x^2 #?

1 Answer
Tony B
Oct 18, 2015

See explanation: Shortcuts given

Explanation:

The #x^2# tells you that it is a quadratic and thus the horse shoe type curve.

#-x^2# tells that it is an inverted horse shoe. This is the standard #(-x)^2#

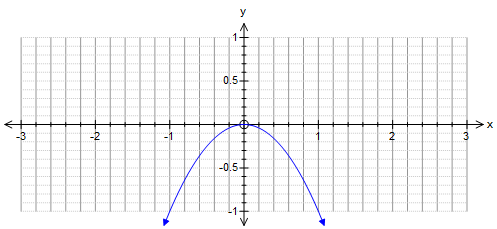
Tony B build

Everything else transforms it in some way.

Multiplying #(-x^2) " or "x^2# by a number greater than one makes the curve steeper.

If the equation was of form #y = -x^2 +bx# then the maximum would be at #x = (-1) times b/2#. However, this equation is of form #y= ax^2 + bx# so we have to do it differently. We would change it to #y=a(x^2 +b/ax)#. Then we may apply the approach of #x =(-1) times 1/2 times b/a#

Lets try it:

Write #y=(-4)x^2 + 16x# as:
#y= -4(x^2 - 4x)#
so the maximum should occur at# (-1/2) times (-4) =2#

Plot of actual graph:
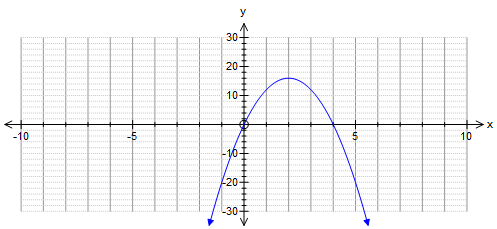
Built by Tony B

Note that what I have shown you is a shortcut to 'completing the square' method

To find where the curve crosses the x-axis substitute y=0 in the original equation. To find the y coordinate of the vertex point substitute the found value of x in the equation. I will let you do that!

Related questions
See all questions in Vertex Form of a Quadratic Equation
Impact of this question
2918 views around the world
You can reuse this answer
Creative Commons License