How do you find all six trigonometric functions of 240 degrees?

2 Answers
Alan P.
May 8, 2015

#240^o# has a reference angle of #60^o# as indicated in the image below. A #60^o# angle is a basic angle from one of the common triangles:
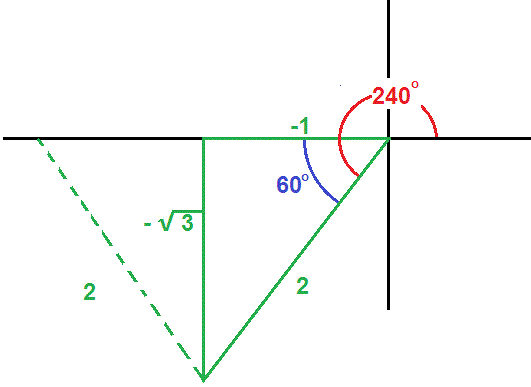
enter image source here

From their definitions:

#sin(240^o) = -sqrt(3)/2#

#cos(240^o) = -1/2#

#tan(240^o) = sqrt(3)#

#csc(240^o) = - 2/sqrt(3)#

#sec(240^o) = -2#

#cot(240^o) = 1/sqrt(3)#

Nghi N.
May 10, 2015

There is another way, using the trig unit circle.

#sin 240 = sin (60 + 180) = -sin 60 = -(sqr3)/2# (trig table)

#cos 240 = cos (60 + 180) = -cos 60 = -1/2#

#tan 240 = sin 240/cos 240 = sqr3#

#cot 240 = = 1/(sqr3) = (sqr3)/3#

#sec 240 = 1/sin 240 = - (2sqr3)/3#

#csc 240 = 1/cos 240 = -2#

Related questions
See all questions in Trigonometric Functions of Any Angle
Impact of this question
38249 views around the world
You can reuse this answer
Creative Commons License