Why can single bonds rotate?

Because the orbitals that form #sigma# bonds are totally symmetric about the internuclear axis, and the properties of vector addition and scalar multiplication of these orbitals is preserved during the bond rotation.

The rotation of a particular single bond does not change the identity of the orbital in question used to make the bond, so the bond itself does not change.

That means we still retain the same molecule after a full #360^@# rotation of the bond as follows:

(Note that rotating double bonds is not possible, and rotating an entire molecule about the internuclear axis is not rotating the bond, but the molecule itself, which doesn't count in any case.)

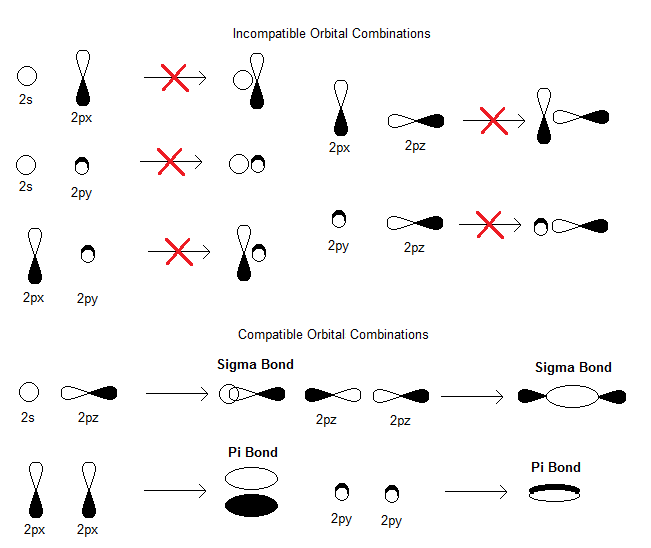
SINGLE BONDS IN RELATION TO HEAD-ON ORBITAL OVERLAP

Single bonds are really the result of one sigma (#sigma#) bond.

So, let's consider two orbitals that are capable of overlapping head-on to generate a #sigma# bond via a linear combination of atomic orbitals (LCAO):

where #sigma_(2p_z)# is the molecular orbital that formed from the linear combination of the two #2p_z# atomic orbitals.

ORBITAL SYMMETRY DICTATES ABILITY TO ROTATE THE BOND

You can see that rotating one of these orbitals about the internuclear axis (the #z# axis) does not change their look at all because they are totally symmetric about the #z# axis.

This is an important feature of a #sigma# bond, because if the orbital looks different upon rotation about the internuclear axis, it would not be what is known as a linear transformation.

A linear transformation preserves the properties of vector addition and scalar multiplication.

This may seem trivial, but it ensures that the two orbitals can continue overlapping as they have been in a LCAO and generate the same molecular orbital upon that overlap.

(It's one of those "do I really need to care" moments in chemistry, but that's the true reason why.)

CONCLUSION

Overall...

If we rotate a single bond about the internuclear axis, if either orbital participating in the bond becomes a different orbital (such as a #2p_x# vs. a #2p_y# orbital), then we are either looking at a different bond or the bond doesn't exist.