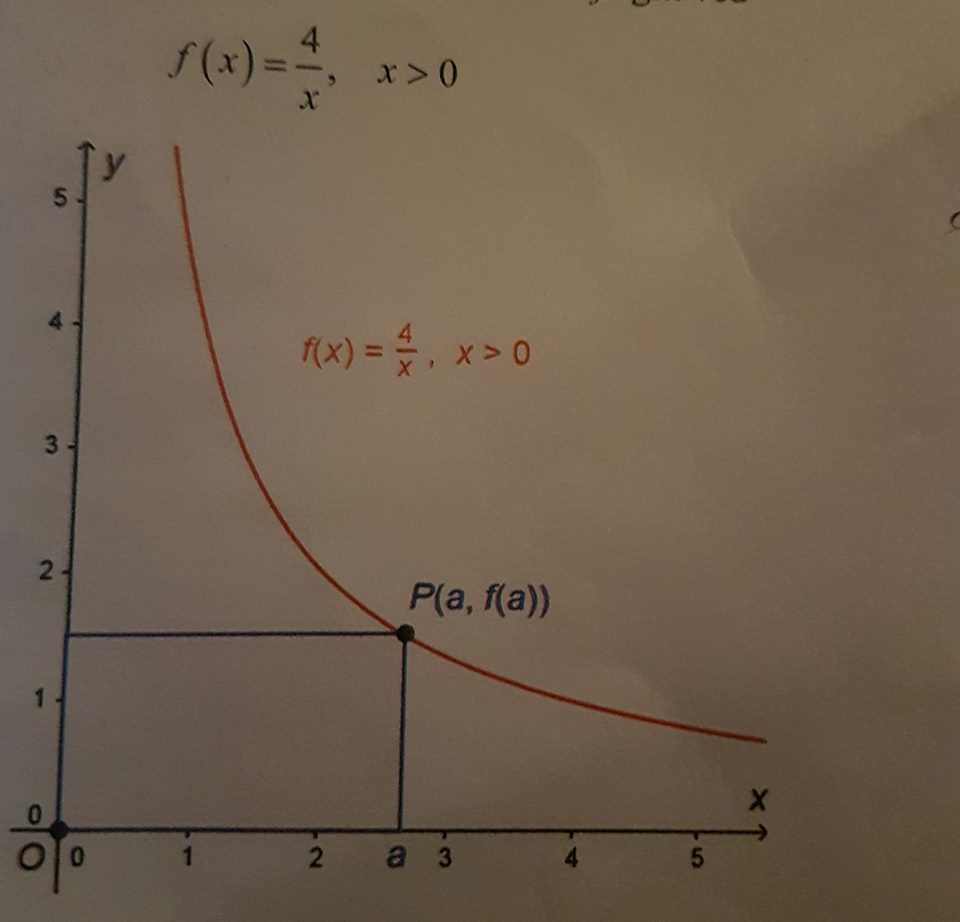
What must (a) be if the perimeter of the figure should be as small as possible?

enter image source here

Should I just guess some numbers and see which gives the smallest perimeter? Or are there any other ways to solve this problem?

1 Answer
Noah G
Apr 22, 2018

#a =2#

Explanation:

The first thing to notice is the height and width of your rectangle.

We know that the first vertex is drawn #a# units from the origin, so the width will be #a#. Then you can see that the height is #f(a)#, or the value of the function at #x = a#. But since the function we are considering is #f(x) = 4/x#, we can see that #f(a) = 4/a#. The expression for perimeter will therefore be

#2(4/a) + 2a = P#

#8/a + 2a = P#

Now differentiate with respect to #P#.

#P' = -8/a^2 + 2#

This will have critical numbers when the derivative equals #0#.

#0 = -8/a^2 + 2#

#-2 = -8/a^2#

#a^2 = 4#

#a = +-2#

The derivative is negative from #(-2, 2)# and positive from #(2, oo)# and #(-oo, 2)#.

Since the transition from negative to positive occurs at #x = 2#, there is a minimum perimeter at #x =2#. This minimum perimeter has value #8/2 + 2(2) = 8# units.

Hopefully this helps!

Related questions
Impact of this question
889 views around the world
You can reuse this answer
Creative Commons License