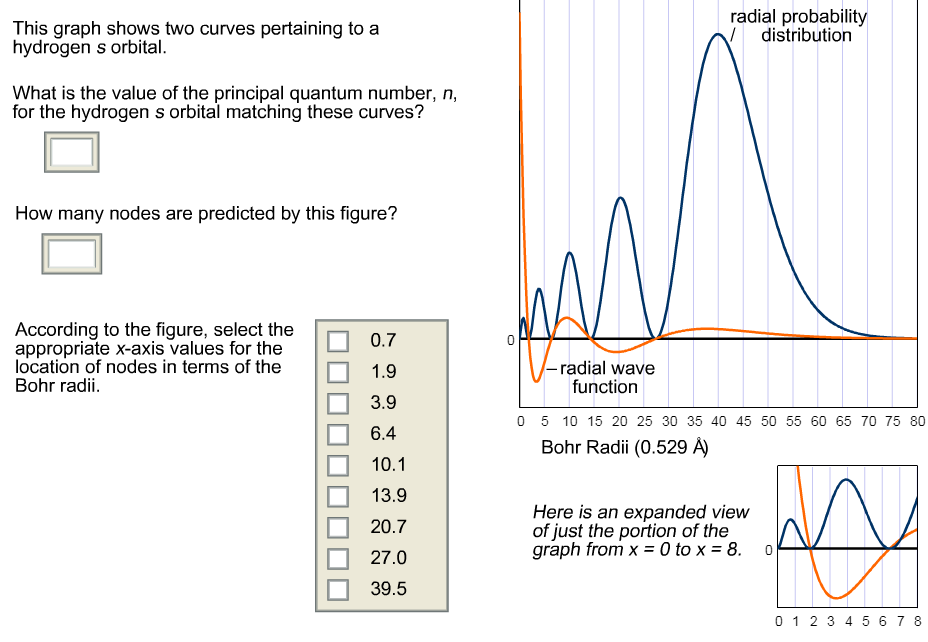
What is the value of the principal quantum number, n, for the hydrogen s orbital matching these curves?

The radial wave function #R_(nl)(r)# (in #color(orange)"orange"#) crosses through zero whenever there are radial nodes, i.e. spherical shells some radial distance away from the nucleus where the electron does not exist.

Alternatively, wherever the radial probability distribution #R_(nl)^2(r)# (in #color(darkblue)"blue"#) touches zero is where the radial node is.

The total number of nodes (of both kinds - radial and angular) tells you what the value of #n# is. For any atomic orbital, the number of nodes in total (of both kinds) is #n - 1#.

Since this is an #bbs# orbital, with zero angular momentum (#l = 0#), it has zero angular nodes.

Therefore, it only has radial nodes, i.e.

#n - 1 = "number of radial nodes in s orbitals"#

I count #4# radial nodes, so #n = 5#, and this is the #bb(5s)# orbital.

For the last part, you don't have to solve any equation. (You can, but you wouldn't have the equation in front of you to use.) Instead, just look, approximate, and find the exact value from the list.

I'm seeing radial nodes at #1.9a_0#, #6.4a_0#, #13.9a_0#, and #27.0a_0#, where #a_0 = "0.0529177 nm"# is the hydrogen atom Bohr radius.