To solve this, we need to know the values of the sin and cos functions at specific angles. One of the simplest ways to look at this is using the unit circle. If we plot a point on the circle that makes the angle theta with the positive x axis in the counter clockwise direction, then the value of cos(theta) is the projection from that point onto the x axis, and the value of sin(theta) is the projection from that point onto the y axis.
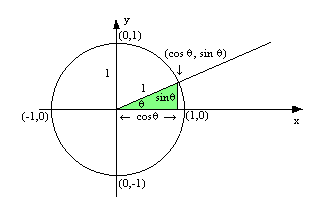
Starting with sin^2(pi//2) = (sin(pi//2))^2 we need to know the sin of the angle theta = pi//2. This is a 90^o angle putting us at the point (0,1) on the unit circle. Therefore, the sin of this angle is 1.
sin^2(pi//2) = 1^2 = 1
The next term is cos(pi). This is an angle of 180^o which puts us at the point (-1,0) on the unit circle, which means that the cos of this angle is -1
cos(pi) = -1
Now we need to put them together:
sin^2(pi//2)-cos(pi) = 1 - (-1) = 2

