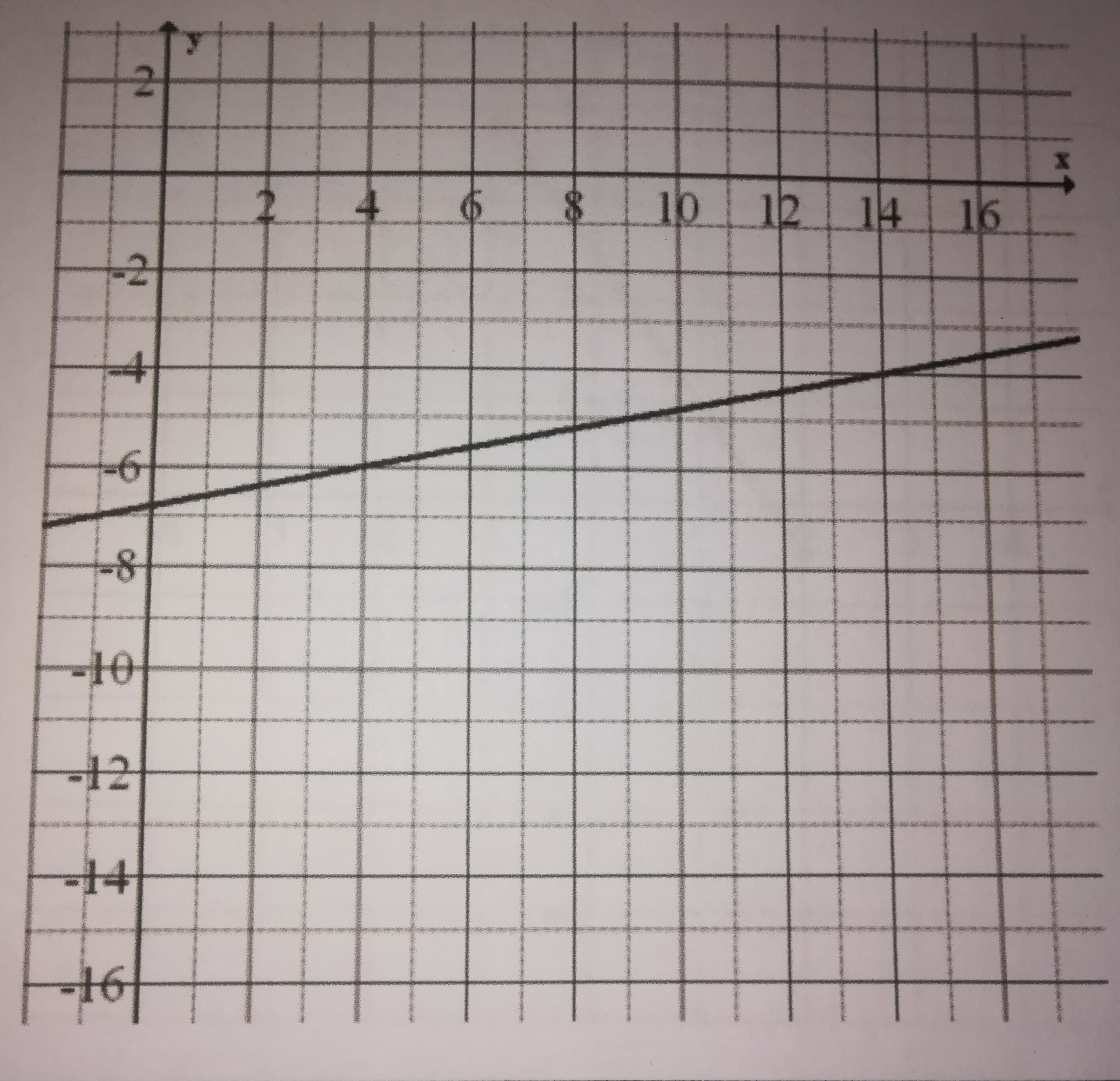
What is the equation of the line shown in the graph in slope-point form?

Practice math sheet

1 Answer
Meave60
Jun 11, 2018

The point-slope form is #y+6=1/5(x-4)# or #y+5=1/5(x-9)#, depending on which point you use. If you solve for #y# to get the slope-intercept form, both equations will convert to #y=1/5x-34/5#.

Explanation:

We have to find the slope first.

I found two points on the line that we can use to find the slope:

#(4,-6)# and #(9,-5)#

Use the slope formula:

#m=(y_2-y_1)/(x_2-x_1)#,

where:

#m# is the slope, and #(x_1,y_1)# is one point, and #(x_2,y_2)# is the other point. I'm going to use #(4,-6)# for #(x_1,y_1)#, and #(9,-5)# for #(x_2,y_2)#.

#m=(-5-(-6))/(9-4)#

#m=1/5#

We could have determined the slope by starting at #(4,-6)# and counting how many spaces to move up and over to get to #(9,-5)#, which would give you #1/5#.

Now that we have the slope, we can determine the point-slope form for this line.

The formula for the point-slope form is:

#y-y_1=m(x-x_1)#

#m=1/5#

I'm going to use #(4,-6)# as the point.

#y-(-6)=1/5(x-4)#

#y+6=1/5(x-4)#

We can also use the second point #(9,-5)#.

#y-(-5)=1/5(x-9)#

#y+5=1/5(x-9)#

If you solve for #y#, which will convert the equation to slope-intercept form, and both equations will come out to #y=1/5x-34/5#.

Related questions
Impact of this question
4472 views around the world
You can reuse this answer
Creative Commons License