The line 3x+2y=6 will divide the quadrilateral formed by the lines #x+y=5 , y-2x=8 , 3y+2x=0 , 4y-x=0# in?

Ans : Two Quadrilaterals

1 Answer
Dean R.
Apr 19, 2018

Graphing it, we see the line divides the quadrilateral into two quadrilaterals.

Explanation:

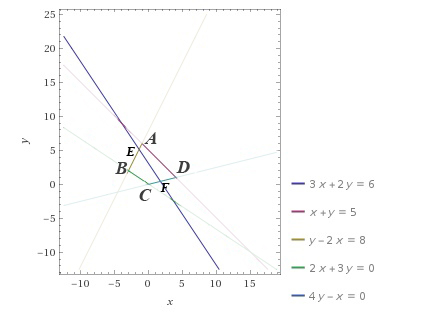
enter image source here
Wolfram Alpha is always good for a picture. I drew on this one. There's actually a fair amount of calculating to verify that the line intersects at the sides, and not the vertices.

Let's find the vertices.

# x+y=5, y-2x=8, 3x=-3, x=-1, y=6, A(-1,6)#
# y-2x=8,3y+2x=0,4y=8,y=2,x=-3, B(-3,2)#
# 3y+2x=0, 4y-x=0,# clearly #x=y=0, C(0,0) #
# 4y-x=0, x+y=5, 5y=5, y=1, x=4, D(4,1) #

Side #AB# has parametric form:
#X=(1-t)A+tB = A + t(B-A)# for #0\le t le 1#
#AB=(-1-2t ,6 -4t )#
#BC=(-3 +3t ,2 - 2t)#
#CD=(4t,t)#
#DA= (4-5t,1+5t)#

Let's find the meets with # 3x+2y=6 #.
# AB: 3(-1-2t)+2(6-4t)=6, t=3/14#
Since #0 < t < 1,# that's a proper meet with side #AB,# not with vertex #A# or #B.#

#BC: 3(-3 + 3t) + 2(2-2t)=6. t=11/5 # outside the segment
#CD: 3(4t)+2t=6, t=3/7 # a proper meet.
#DA: 3(4-5t)+2(1+5t)=6, t=8/5 # not a segment meet

For completeness,

# E= 11/14 A + 3/14 B = (- 10/7, 36/7 ) #

# F=(4t,t)=(12/7, 3/7) #

Related questions
Impact of this question
2417 views around the world
You can reuse this answer
Creative Commons License