The half-life of a first-order reaction is 1.5 hours. How much time is needed for 94% of the reactant to change to product?

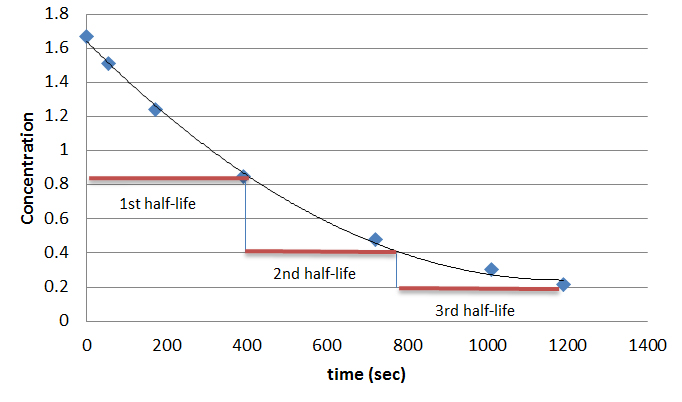
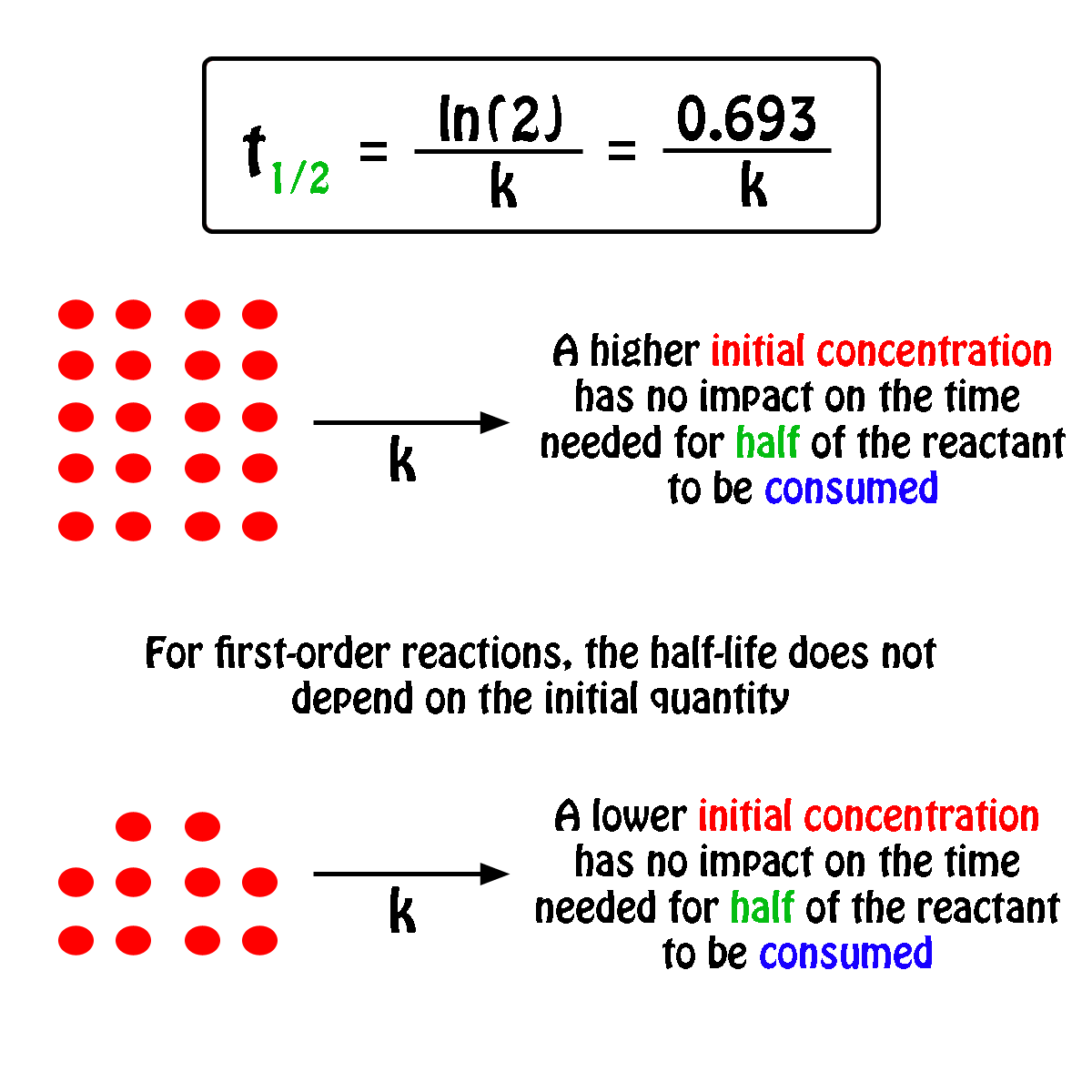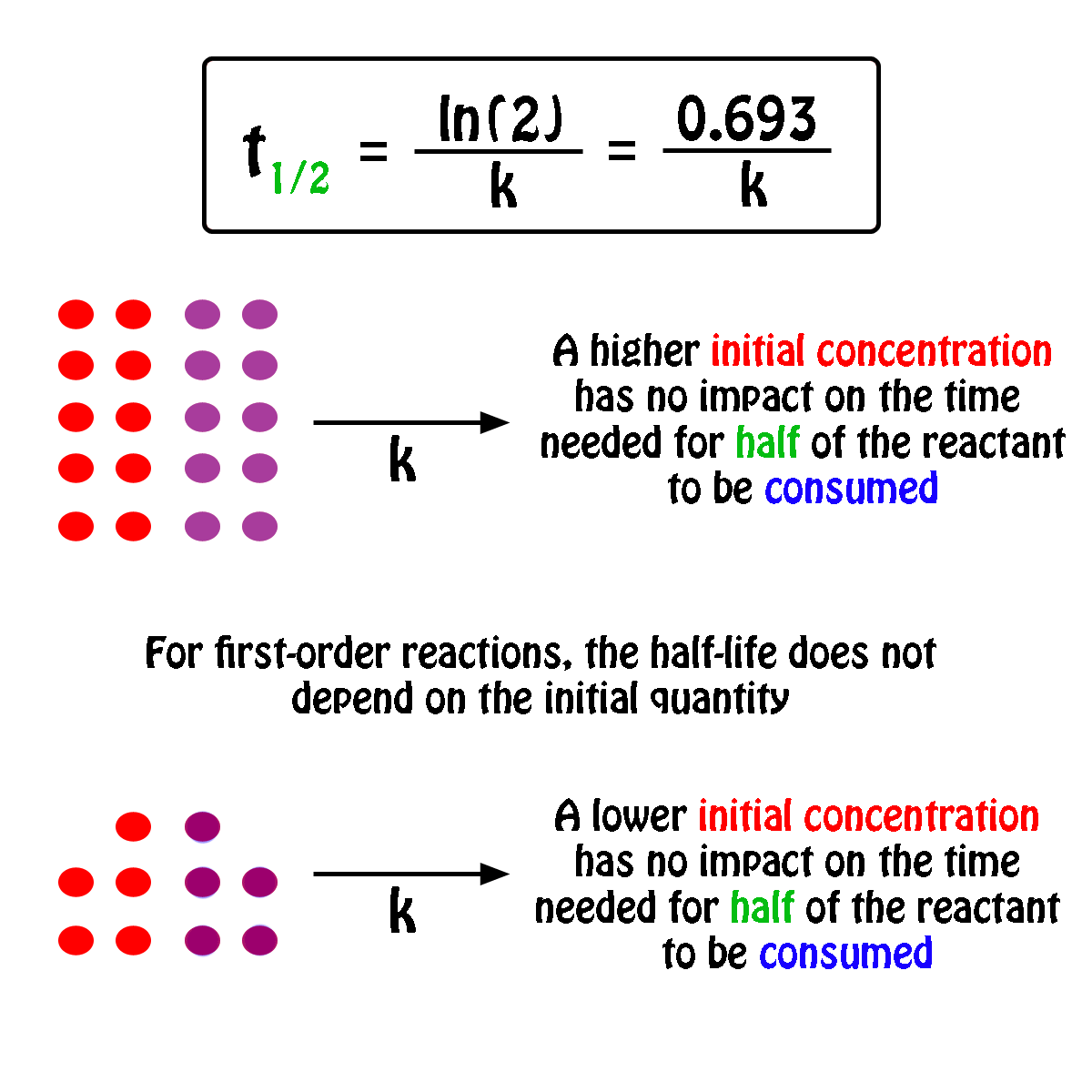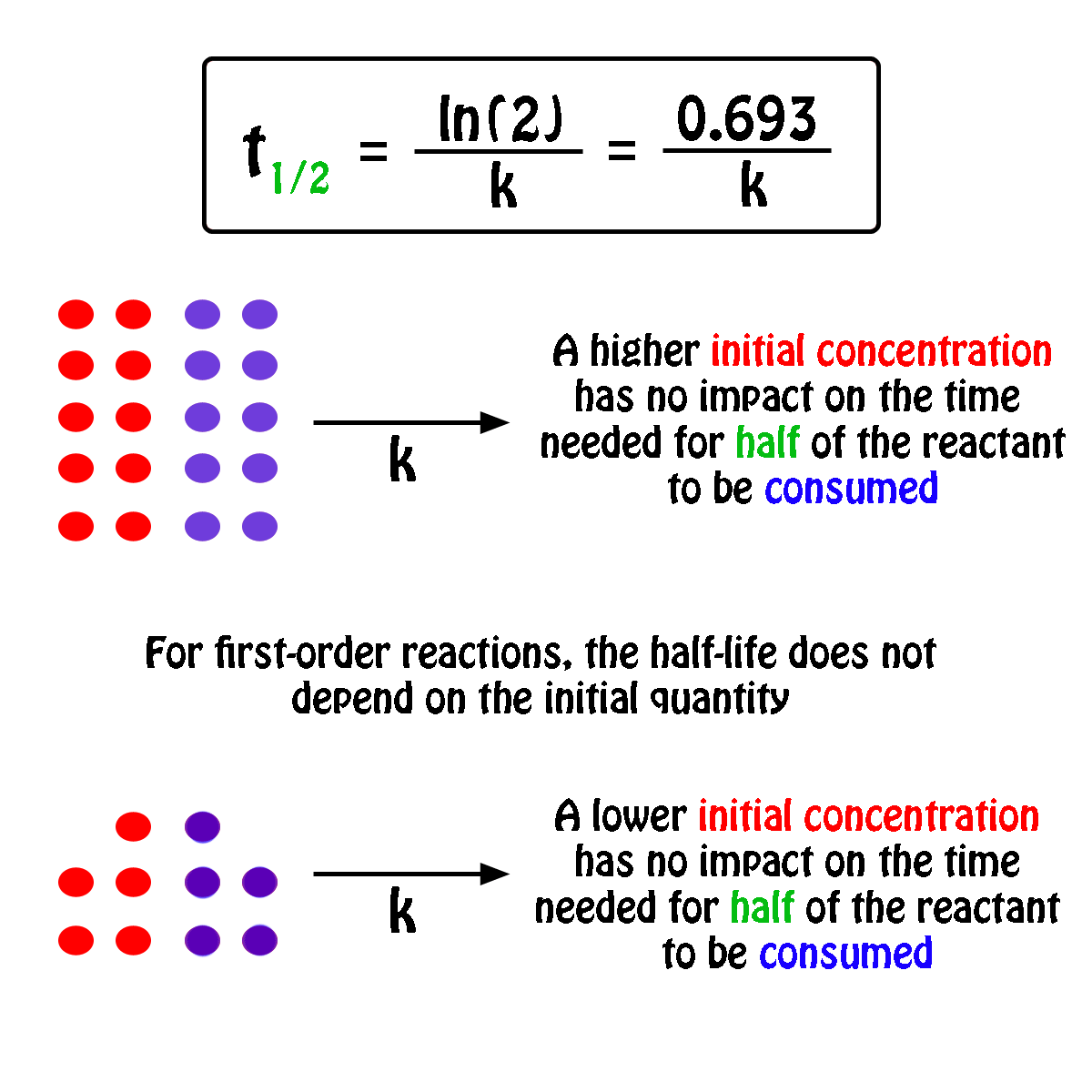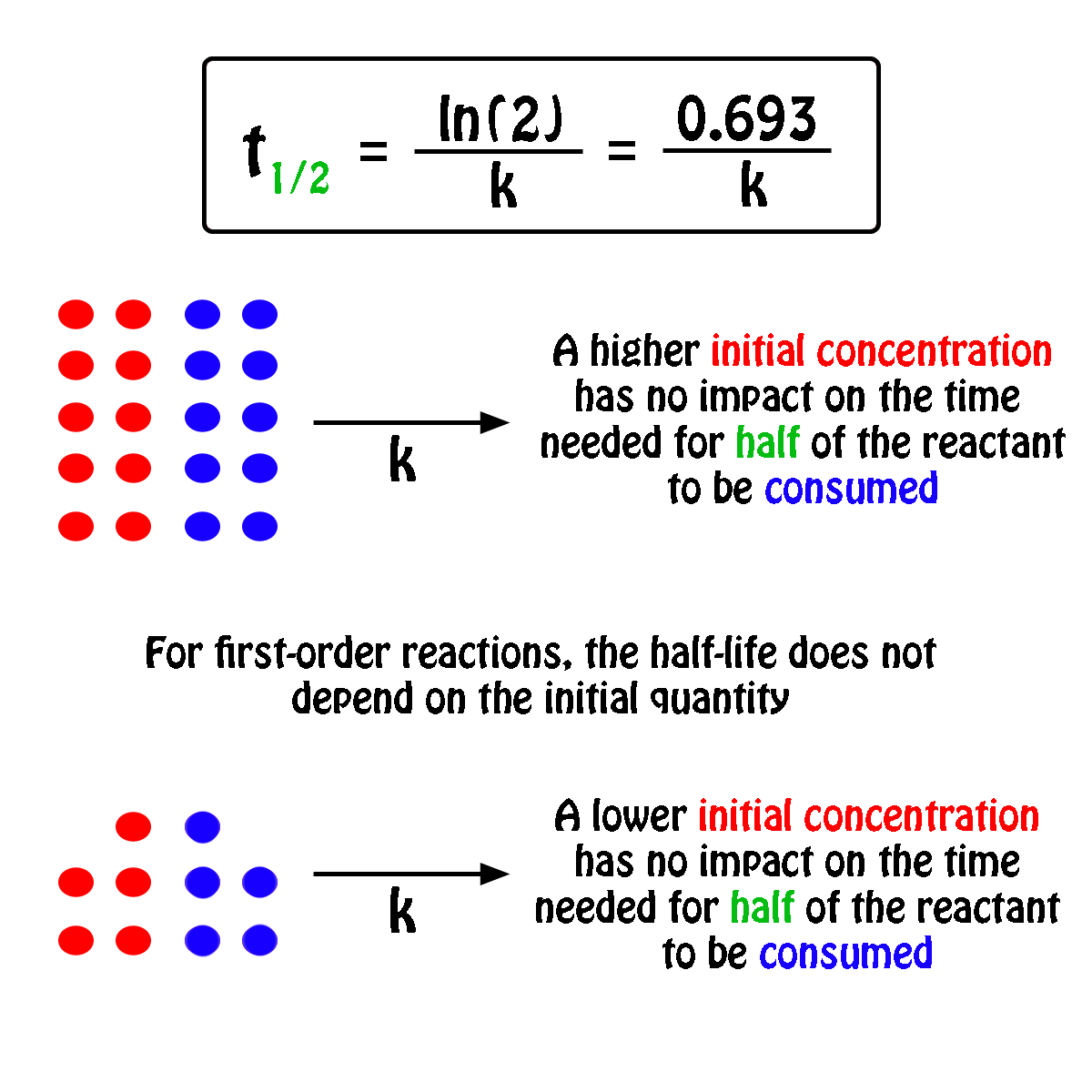
The thing to remember about first-order reactions is that their half-life is independent of the initial concentration of the reactant.

In other words, it doesn't matter how much reactant you have, the half-life of the reaction, i.e. the time needed for half of the initial concentration of the reactant to be consumed, will be constant.

Mathematically, you can express this as

#["A"]_t = ["A"]_0 * e^(-kt)#

Here

• #["A"]_t# represents the concentration of the reactant after a time #t#
• #["A"]_0# represents the initial concentration of the reactant
• #k# is the rate constant

Now, start by isolating #t# on one side of the equation. To do that, take the natural log of both sides

#ln(["A"]_t) = ln(["A"]_0 * e^(-kt))#

This will get you

#ln(["A"]) - ln(["A"]_0) = - kt * ln(e)#

#ln(["A"]_0) - ln(["A"]) = kt#

Finally, you have

#t = ln (["A"]_0/["A"]_t)/k " " " "color(darkorange)("(*)")#

Now, you know that after the passing of one half-life, #t_"1/2"#, the concentration of the reactant is reduced to half of its initial value.

#["A"]_ t = ["A"]_ 0/2 " "->" " "after t"_"1/2"#

This means that you have

#ln(["A"]_0/["A"]_t) = ln(color(red)(cancel(color(black)(["A"]_0)))/(color(red)(cancel(color(black)(["A"]_0)))/2)) = ln(2)#

and so

#t_"1/2" = ln(2)/k#

This means that the rate constant of the reaction is equal to

#k = ln(2)/t_"1/2"#

In your case, you have

#k = ln(2)/"1.5 h" = "0.462 h"^(-1)#

Finally, you know that in order for #94%# of the reactant to be consumed, you need to have

#["A"]_t = (1 - 94/100) * ["A"]_0#

This basically means that when #94%# of the reactant is consumed, you are left with #6%# of what you started with.

Plug this into equation #color(darkorange)("(*)")# to find

#t = ln( (color(red)(cancel(color(black)(["A"]_0))))/(3/100 * color(red)(cancel(color(black)(["A"]_0)))))/"0.462 h"^(-1) = color(darkgreen)(ul(color(black)("7.6 h")))#

The answer is rounded to two sig figs, the number of sig figs you have for the half-life of the reaction.