Solve 22 and 24 number.it is simple differentiation but tell me what is mean by f(x) and g(x) here and how solve it.i am very worried.?
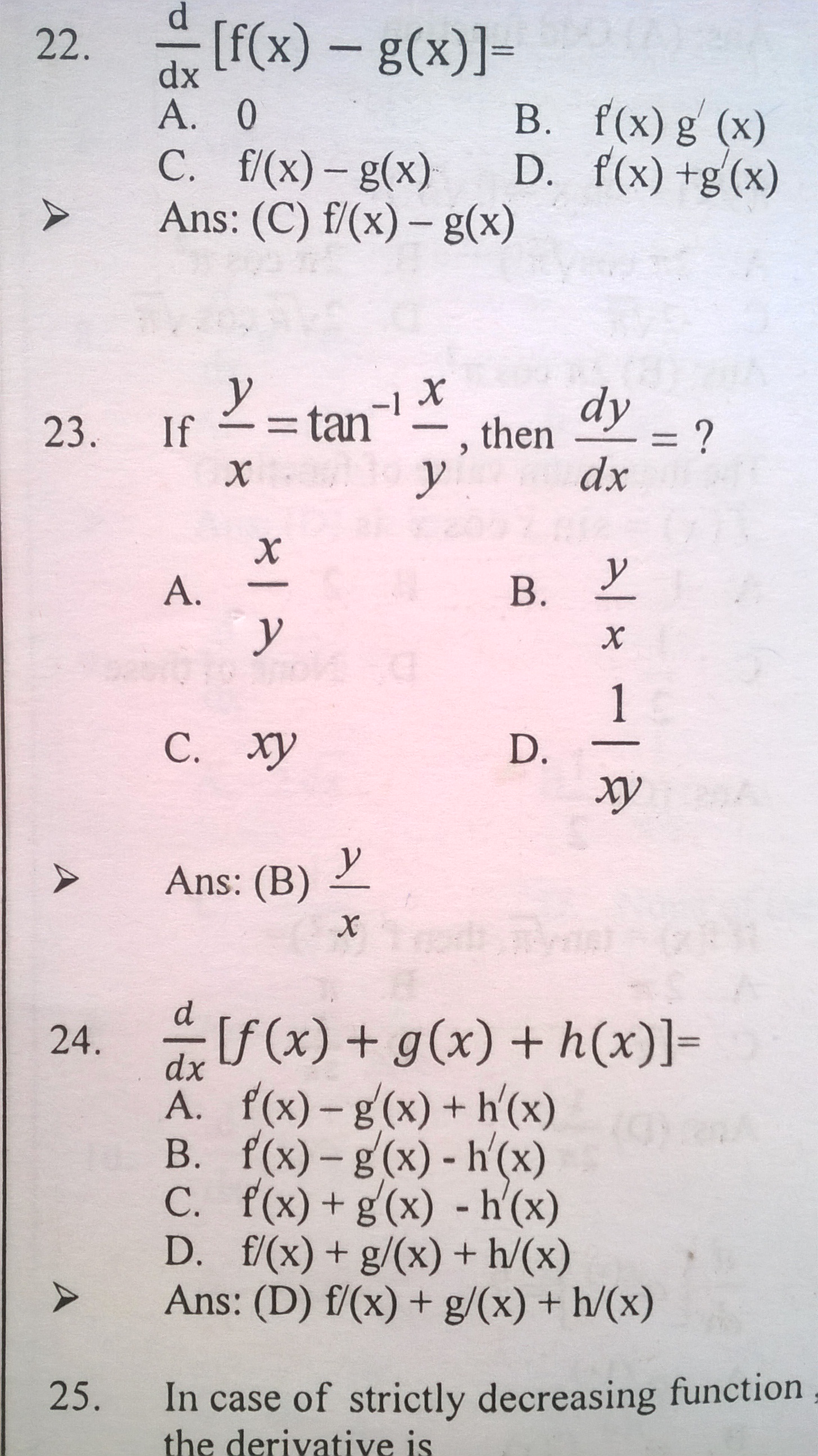
3 Answers
See explanation.
Explanation:
Questions 22 and 24 are the basic properties of derivatives.
22 says that the derivative of a difference of 2 functions is the difference of the functions' derivatives
24 says that the derivative of a sum of 3 functions is the sum of the functions' derivatives.
In these questions
Please see below.
Explanation:
I think that you are asking about the notation
We use this notation for a function.
One way to think about a function is simply as another name for
So instead of writing
#y = x^2+3#
We can write
#f(x) = x^2+3#
We read this "f of x equals x squared plus 3".
#f# is the name of the function and#f(x)# is a name for#y# .
Instead of writing:
If
#y = x^2+3# , find#y# when#x = 2#
We can write
If
#f(x) = x^2+3# , find#f(3)#
We read#f(3)# as "f of 3# and, since # (3)^2+3 = 9#, we say "f of 3 is [or equals] 9".
A function is like an operation performed on some input. It takes an input and gives an output. Some of the algebra operations and some calculator buttons are (simple) functions.
With
Instead of saying
Find
#dy/dx# when#x = 5#
we can say
Find
#f'(5)# ("find f prime of 5")
You can read more about functions at Socratic under the Subject: Algebra.
Use the Subject pull-down list above. Select Algebra.
Then when Algebra loads, select Topics. You can look for topics about functions.
On a personal note
Many students are now taught about functions when they learn algebra. The first time I saw a function or the word "function" was in my first calculus class.
For a long time I thought of functions as I have described them.
There's more to it than that, but it gets you going in the right direction.
Question 22 could be rewritten as follows.
With
what is
#d/dx(y-z)# ?
Answer (C.)

