Put this function in vertex form by completing the square?

Question

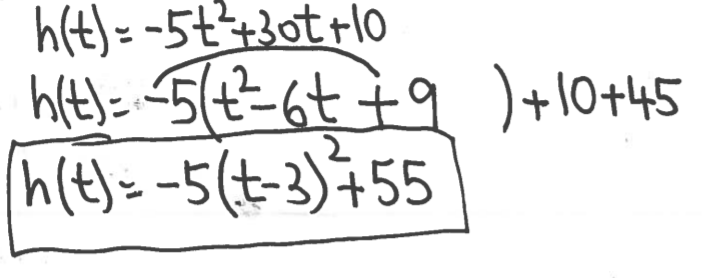
I'm really confused on how they completed the square. Can someone please do a step by step walkthrough on how they solved this problem?

1972 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-12-14T03:31:13

h(t)=5(t-3)^2 +55

h(t)=-5t^2+30t+10
We want equation in this form y={A(x-B)^2}+C

So we have to change -5t^2+30t+10 into
{A(x-B)^2}+C

Now
-5t^2+30t+10
Taking 5 common we get
-5(t^2-6t-2)
-5(t^2-23t+3×3-3×3-2)
Hint
(a-b)^2= a^2-2ab+b^2

So now
-5{(t^2-2×3×t+3^2)-11}
-5{(t-3)^2 -11}
-5*(t-3)^2 +55

That gives
h(t)=-5*(t-3)^2 +55