In the table below, fill in as many y values as you can if you know that f is an even function, and g is an odd function?
Enter the letter "n" if you cannot fill in a cell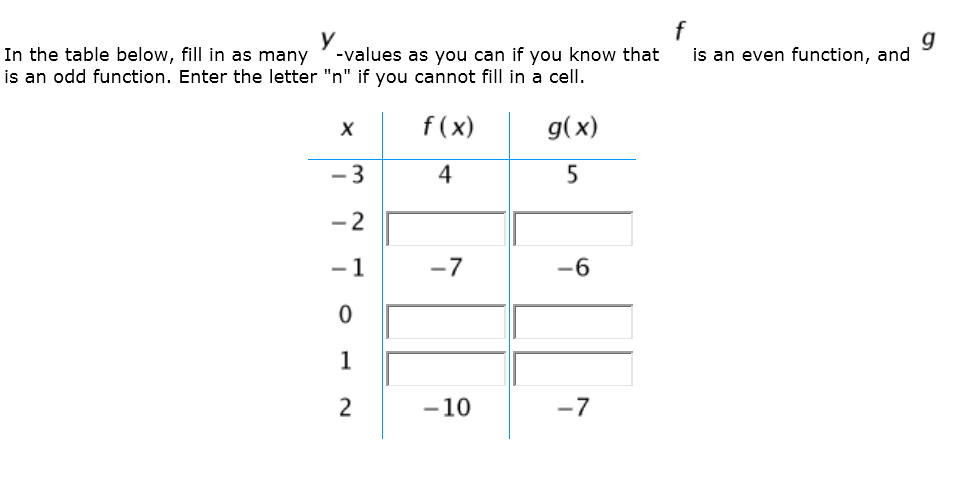
How would I start this problem?
Enter the letter "n" if you cannot fill in a cell
How would I start this problem?
1 Answer
Jul 28, 2018
Kindly refer to Explanation.
Explanation:
Since
Next,
Observe that, for any odd function like
The rest are n.
